Hostname: page-component-586b7cd67f-l7hp2 Total loading time: 0 Render date: 2024-11-22T05:58:03.493Z Has data issue: false hasContentIssue false
  • English
  • Français

Stability and Turán Numbers of a Class of Hypergraphs via Lagrangians

Part of: Graph theory

Published online by Cambridge University Press:  29 March 2017

AXEL BRANDT ,
DAVID IRWIN  and
TAO JIANG
AXEL BRANDT
Affiliation:
Department of Mathematics and Computer Science, Davidson College, Davidson, NC 28035, USA (e-mail: [email protected])
DAVID IRWIN
Affiliation:
Department of Mathematics, Ohio State University, Columbus, OH 43210, USA (e-mail: [email protected])
TAO JIANG
Affiliation:
Department of Mathematics, Miami University, Oxford, OH 45056, USA (e-mail: [email protected])
Get access Rights & Permissions [Opens in a new window]

Abstract

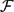Given a family of r-uniform hypergraphs ${\cal F}$ (or r-graphs for brevity), the Turán number ex(n,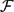${\cal F})$ of 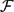${\cal F}$ is the maximum number of edges in an r-graph on n vertices that does not contain any member of 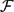${\cal F}$. A pair {u,v} is covered in a hypergraph G if some edge of G contains {u, v}. Given an r-graph F and a positive integer p ⩾ n(F), where n(F) denotes the number of vertices in F, let HFp denote the r-graph obtained as follows. Label the vertices of F as v1,. . .,vn(F). Add new vertices vn(F)+1,. . .,vp. For each pair of vertices vi, vj not covered in F, add a set Bi,j of r − 2 new vertices and the edge {vi, vj} ∪ Bi,j, where the Bi,j are pairwise disjoint over all such pairs {i, j}. We call HFp the expanded p-clique with an embedded F. For a relatively large family of F, we show that for all sufficiently large n, ex(n,HFp) = |Tr(n, p − 1)|, where Tr(n, p − 1) is the balanced complete (p − 1)-partite r-graph on n vertices. We also establish structural stability of near-extremal graphs. Our results generalize or strengthen several earlier results and provide a class of hypergraphs for which the Turán number is exactly determined (for large n).

MSC classification

Primary: 05C65: Hypergraphs
Secondary: 05C35: Extremal problems
Type
Paper
Information
Combinatorics, Probability and Computing , Volume 26 , Issue 3 , May 2017 , pp. 367 - 405
Copyright
Copyright © Cambridge University Press 2017 

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

[1] Ajtai, M., Komlós, J., Simonovits, M. and Szemerédi, E. Unpublished. The solution of the Erdos-Sos conjecture for large trees, in preparation.Google Scholar
[2] Bollobás, B. (1974) Three-graphs without two triples whose symmetric difference is contained in a third. Discrete Math. 8 21–24.CrossRefGoogle Scholar
[3] Erdős, P. (1964) On extremal problems of graphs and generalized graphs. Israel J. Math. 2 183–190.CrossRefGoogle Scholar
[4] Erdős, P. (1965) A problem on independent r-tuples. Ann. Univ. Sci. Budapest 8 93–95.Google Scholar
[5] Erdős, P. and Simonovits, M. (1966) A limit theorem in graph theory. Studia. Sci. Math. Hungar. 1 51–57.Google Scholar
[6] Frankl, P. (2013) Improved bounds for Erdős' matching conjecture. J. Combin. Theory Ser. A 120 1068–1072.CrossRefGoogle Scholar
[7] Frankl, P. and Füredi, Z. (1983) A new generalization of the Erdős–Ko–Rado theorem. Combinatorica 3 341–349.CrossRefGoogle Scholar
[8] Frankl, P. and Füredi, Z. (1989) Extremal problems whose solutions are the blowups of the small Witt-designs. J. Combin. Theory Ser. A 52 129–147.CrossRefGoogle Scholar
[9] Füredi, Z., Pikhurko, O. and Simonovits, M. (2005) On triple systems with independent neighbourhoods. Combin. Probab. Comput. 14 795–813.CrossRefGoogle Scholar
[10] Hefetz, D. and Keevash, P. (2013) A hypergraph Turán theorem via Lagrangians of intersecting families. J. Combin. Theory Ser. A 120 2020–2038.CrossRefGoogle Scholar
[11] Jiang, T., Peng, Y. and Wu, B. Turán numbers of extensions of some sparse hypergraphs via Lagrangians, in preparation. arXiv:1609.08983 Google Scholar
[12] Katona, G. O. H. (1974) Extremal problems for hypergraphs. In Combinatorics (Hall, M. Jr and van Lint, J. H., eds), Vol. 16 of the NATO Advanced Study Institutes series, Springer pp. 215–244.Google Scholar
[13] Keevash, P. (2011) Hypergraph Turán Problems, Surveys in Combinatorics, Cambridge University Press, pp. 83–140.Google Scholar
[14] Keevash, P. and Mubayi, D. (2004) Stability theorems for cancellative hypergraphs. J. Combin. Theory Ser. B 92 163–175.CrossRefGoogle Scholar
[15] Mubayi, D. (2006) A hypergraph extension of Turán's theorem. J. Combin. Theory Ser. B 96 122–134.CrossRefGoogle Scholar
[16] Mubayi, D. and Pikhurko, O. (2007) A new generalization of Mantel's theorem to k-graphs. J. Combin. Theory Ser. B 97 669–678.CrossRefGoogle Scholar
[17] Motzkin, T. S. and Straus, E. G. (1965) Maxima for graphs and a new proof of a theorem of Turán. Canad. J. Math. 17 533–540.CrossRefGoogle Scholar
[18] Norin, S. and Yepremyan, L. (2017) Turán number of generalized triangles. J. Combin. Theory Ser. A 146 312–343.CrossRefGoogle Scholar
[19] Norin, S. and Yepremyan, L. Turán numbers of extensions, in preparation. arXiv:1510.04689 Google Scholar
[20] Pikhurko, O. (2008) An exact Turán result for the generalized triangle. Combinatorica 28 187–208.CrossRefGoogle Scholar
[21] Pikhurko, O. (2013) Exact computation of the hypergraph Turán function for expanded complete 2-graphs. J. Combin. Theory Ser. B 103 220–225.CrossRefGoogle Scholar
[22] Shearer, J. (1996) A new construction for cancellative families of sets. Electron. J. Combin. 3 R15.CrossRefGoogle Scholar
[23] Sidorenko, A. F. (1987) On the maximal number of edges in a homogeneous hypergraph that does not contain prohibited subgraphs. Mat. Zametki 41 433–455.Google Scholar
[24] Sidorenko, A. F. (1989) Asymptotic solution for a new class of forbidden r-graphs. Combinatorica 9 207–215.CrossRefGoogle Scholar
[25] Simonovits, M. (1968) A method for solving extremal problems in graph theory. In Theory of Graphs: Proc. Coll. Tihany, Hungary (Erdős, P. and Katona, G., eds), Academic Press, pp. 279–319.Google Scholar
[26] Yepremyan, L. (2016) PhD dissertation, McGill University.Google Scholar