Published online by Cambridge University Press: 26 May 2021
Let 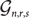 $${{\mathcal G}_{n,r,s}}$$ denote a uniformly random r-regular s-uniform hypergraph on the vertex set {1, 2, … , n}. We establish a threshold result for the existence of a spanning tree in
$${{\mathcal G}_{n,r,s}}$$ denote a uniformly random r-regular s-uniform hypergraph on the vertex set {1, 2, … , n}. We establish a threshold result for the existence of a spanning tree in 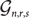 $${{\mathcal G}_{n,r,s}}$$, restricting to n satisfying the necessary divisibility conditions. Specifically, we show that when s ≥ 5, there is a positive constant ρ(s) such that for any r ≥ 2, the probability that
$${{\mathcal G}_{n,r,s}}$$, restricting to n satisfying the necessary divisibility conditions. Specifically, we show that when s ≥ 5, there is a positive constant ρ(s) such that for any r ≥ 2, the probability that 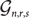 $${{\mathcal G}_{n,r,s}}$$ contains a spanning tree tends to 1 if r > ρ(s), and otherwise this probability tends to zero. The threshold value ρ(s) grows exponentially with s. As
$${{\mathcal G}_{n,r,s}}$$ contains a spanning tree tends to 1 if r > ρ(s), and otherwise this probability tends to zero. The threshold value ρ(s) grows exponentially with s. As 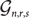 $${{\mathcal G}_{n,r,s}}$$ is connected with probability that tends to 1, this implies that when r ≤ ρ(s), most r-regular s-uniform hypergraphs are connected but have no spanning tree. When s = 3, 4 we prove that
$${{\mathcal G}_{n,r,s}}$$ is connected with probability that tends to 1, this implies that when r ≤ ρ(s), most r-regular s-uniform hypergraphs are connected but have no spanning tree. When s = 3, 4 we prove that 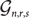 $${{\mathcal G}_{n,r,s}}$$ contains a spanning tree with probability that tends to 1, for any r ≥ 2. Our proof also provides the asymptotic distribution of the number of spanning trees in
$${{\mathcal G}_{n,r,s}}$$ contains a spanning tree with probability that tends to 1, for any r ≥ 2. Our proof also provides the asymptotic distribution of the number of spanning trees in 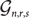 $${{\mathcal G}_{n,r,s}}$$ for all fixed integers r, s ≥ 2. Previously, this asymptotic distribution was only known in the trivial case of 2-regular graphs, or for cubic graphs.
$${{\mathcal G}_{n,r,s}}$$ for all fixed integers r, s ≥ 2. Previously, this asymptotic distribution was only known in the trivial case of 2-regular graphs, or for cubic graphs.
Supported by the Australian Research Council Discovery Project DP190100977.
Supported by the Australian Research Council Discovery Early Career Researcher Award DE200101045.