Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by Crossref.
DONG, F. M.
and
KOH, K. M.
2008.
Bounds For The Real Zeros of Chromatic Polynomials.
Combinatorics, Probability and Computing,
Vol. 17,
Issue. 6,
p.
749.
Salas, Jesús
and
Sokal, Alan D.
2009.
Transfer Matrices and Partition-Function Zeros for Antiferromagnetic Potts Models.
Journal of Statistical Physics,
Vol. 135,
Issue. 2,
p.
279.
Bissacot, Rodrigo
Fernández, Roberto
and
Procacci, Aldo
2010.
On the Convergence of Cluster Expansions for Polymer Gases.
Journal of Statistical Physics,
Vol. 139,
Issue. 4,
p.
598.
Chang, Shu-Chiuan
and
Shrock, Robert
2010.
Weighted Graph Colorings.
Journal of Statistical Physics,
Vol. 138,
Issue. 1-3,
p.
496.
Salas, Jesús
and
Sokal, Alan D.
2011.
Transfer Matrices and Partition-Function Zeros for Antiferromagnetic Potts Models VI. Square Lattice with Extra-Vertex Boundary Conditions.
Journal of Statistical Physics,
Vol. 144,
Issue. 5,
p.
1028.
Ellis-Monaghan, Joanna A.
and
Merino, Criel
2011.
Structural Analysis of Complex Networks.
p.
219.
Fadnavis, Sukhada
2012.
On Brenti’s conjecture about the log-concavity of the chromatic polynomial.
European Journal of Combinatorics,
Vol. 33,
Issue. 8,
p.
1842.
Ndreca, Sokol
Procacci, Aldo
and
Scoppola, Benedetto
2012.
Improved bounds on coloring of graphs.
European Journal of Combinatorics,
Vol. 33,
Issue. 4,
p.
592.
Jackson, Bill
Procacci, Aldo
and
Sokal, Alan D.
2013.
Complex zero-free regions at large |q| for multivariate Tutte polynomials (alias Potts-model partition functions) with general complex edge weights.
Journal of Combinatorial Theory, Series B,
Vol. 103,
Issue. 1,
p.
21.
Morais, Thiago
and
Procacci, Aldo
2013.
Continuous Particles in the Canonical Ensemble as an Abstract Polymer Gas.
Journal of Statistical Physics,
Vol. 151,
Issue. 5,
p.
830.
Morais, Thiago
Procacci, Aldo
and
Scoppola, Benedetto
2014.
On Lennard–Jones Type Potentials and Hard-Core Potentials with an Attractive Tail.
Journal of Statistical Physics,
Vol. 157,
Issue. 1,
p.
17.
Fadnavis, Sukhada
2015.
A note on the shameful conjecture.
European Journal of Combinatorics,
Vol. 47,
Issue. ,
p.
115.
Brown, Jason
and
Erey, Aysel
2015.
New bounds for chromatic polynomials and chromatic roots.
Discrete Mathematics,
Vol. 338,
Issue. 11,
p.
1938.
Royle, Gordon F.
and
Sokal, Alan D.
2015.
Linear Bound in Terms of Maxmaxflow for the Chromatic Roots of Series-Parallel Graphs.
SIAM Journal on Discrete Mathematics,
Vol. 29,
Issue. 4,
p.
2117.
Liu, Jingcheng
Sinclair, Alistair
and
Srivastava, Piyush
2019.
A Deterministic Algorithm for Counting Colorings with 2-Delta Colors.
p.
1380.
Liu, Jingcheng
Sinclair, Alistair
and
Srivastava, Piyush
2022.
Correlation Decay and Partition Function Zeros: Algorithms and Phase Transitions.
SIAM Journal on Computing,
p.
FOCS19-200.
Procacci, Aldo
2022.
Virial series for a system of classical particles interacting through a pair potential with negative minimum.
Reviews in Mathematical Physics,
Vol. 34,
Issue. 03,
Bencs, Ferenc
Huijben, Jeroen
and
Regts, Guus
2024.
Approximating the chromatic polynomial is as hard as computing it exactly.
computational complexity,
Vol. 33,
Issue. 1,
Jenssen, Matthew
Patel, Viresh
and
Regts, Guus
2024.
Improved bounds for the zeros of the chromatic polynomial via Whitney's Broken Circuit Theorem.
Journal of Combinatorial Theory, Series B,
Vol. 169,
Issue. ,
p.
233.


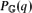 of a finite graph
of a finite graph  of maximal degree Δ is free of zeros for |
of maximal degree Δ is free of zeros for |