No CrossRef data available.
Article contents
On the Ramsey numbers of daisies II
Published online by Cambridge University Press: 18 September 2024
Abstract
A 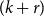 $(k+r)$-uniform hypergraph
$(k+r)$-uniform hypergraph 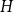 $H$ on
$H$ on 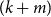 $(k+m)$ vertices is an
$(k+m)$ vertices is an 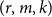 $(r,m,k)$-daisy if there exists a partition of the vertices
$(r,m,k)$-daisy if there exists a partition of the vertices 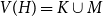 $V(H)=K\cup M$ with
$V(H)=K\cup M$ with  $|K|=k$,
$|K|=k$, 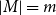 $|M|=m$ such that the set of edges of
$|M|=m$ such that the set of edges of 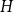 $H$ is all the
$H$ is all the 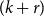 $(k+r)$-tuples
$(k+r)$-tuples 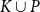 $K\cup P$, where
$K\cup P$, where 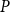 $P$ is an
$P$ is an  $r$-tuple of
$r$-tuple of 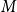 $M$. We obtain an
$M$. We obtain an  $(r-2)$-iterated exponential lower bound to the Ramsey number of an
$(r-2)$-iterated exponential lower bound to the Ramsey number of an 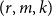 $(r,m,k)$-daisy for
$(r,m,k)$-daisy for 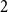 $2$-colours. This matches the order of magnitude of the best lower bounds for the Ramsey number of a complete
$2$-colours. This matches the order of magnitude of the best lower bounds for the Ramsey number of a complete  $r$-graph.
$r$-graph.
- Type
- Paper
- Information
- Copyright
- © The Author(s), 2024. Published by Cambridge University Press
Footnotes
The author was supported by NSF grant DMS 1764385 and US Air Force grant FA9550-23-1-0298.



