Hostname: page-component-586b7cd67f-gb8f7 Total loading time: 0 Render date: 2024-11-25T04:38:15.219Z Has data issue: false hasContentIssue false
  • English
  • Français

On Colourings of Hypergraphs Without Monochromatic Fano Planes

Published online by Cambridge University Press:  01 September 2009

HANNO LEFMANN ,
YURY PERSON ,
VOJTĚCH RÖDL  and
MATHIAS SCHACHT
HANNO LEFMANN
Affiliation:
Fakultät für Informatik, Technische Universität Chemnitz, D-09107 Chemnitz, Germany (e-mail: [email protected])
YURY PERSON
Affiliation:
Institut für Informatik, Humboldt-Universität zu Berlin, D-10099 Berlin, Germany (e-mail: [email protected], [email protected])
VOJTĚCH RÖDL
Affiliation:
Department of Mathematics and Computer Science, Emory University, Atlanta, GA 30322, USA (e-mail: [email protected])
MATHIAS SCHACHT
Affiliation:
Institut für Informatik, Humboldt-Universität zu Berlin, D-10099 Berlin, Germany (e-mail: [email protected], [email protected])
Get access Rights & Permissions [Opens in a new window]

Abstract

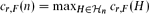For k-uniform hypergraphs F and H and an integer r, let cr,F(H) denote the number of r-colourings of the set of hyperedges of H with no monochromatic copy of F, and let , where the maximum runs over all k-uniform hypergraphs on n vertices. Moreover, let ex(n,F) be the usual extremal or Turán function, i.e., the maximum number of hyperedges of an n-vertex k-uniform hypergraph which contains no copy of F.

For complete graphs F = Kℓ and r = 2, Erdős and Rothschild conjectured that c2,Kℓ(n) = 2ex(n,Kℓ). This conjecture was proved by Yuster for ℓ = 3 and by Alon, Balogh, Keevash and Sudakov for arbitrary ℓ. In this paper, we consider the question for hypergraphs and show that, in the special case when F is the Fano plane and r = 2 or 3, then cr,F(n) = rex(n,F), while cr,F(n) ≫ rex(n,F) for r ≥ 4.

Type
Paper
Information
Combinatorics, Probability and Computing , Volume 18 , Issue 5: New Directions in Algorithms, Combinatorics and Optimization , September 2009 , pp. 803 - 818
Copyright
Copyright © Cambridge University Press 2009

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

[1]Alon, N., Balogh, J., Keevash, P. and Sudakov, B. (2004) The number of edge colorings with no monochromatic cliques. J. London Math. Soc. (2) 70 273–288.CrossRefGoogle Scholar
[2]Balogh, J. (2006) A remark on the number of edge colorings of graphs. Europ. J. Combin. 27 565–573.CrossRefGoogle Scholar
[3]Balogh, J., Bollobás, B. and Simonovits, M. (2004) The number of graphs without forbidden subgraphs. J. Combin. Theory Ser. B 91 1–24.CrossRefGoogle Scholar
[4]Balogh, J., Bollobás, B. and Simonovits, M. (2009) The typical structure of graphs without given excluded subgraphs. Random Struct. Alg. 34 305–318.CrossRefGoogle Scholar
[5]Chung, F. R. K. (1991) Regularity lemmas for hypergraphs and quasi-randomness. Random Struct. Alg. 2 241–252.CrossRefGoogle Scholar
[6]Erdős, P. (1974) Some new applications of probability methods to combinatorial analysis and graph theory. In Proc. Fifth Southeastern Conference on Combinatorics, Graph Theory and Computing (Florida Atlantic University, Boca Raton 1974), pp. 39–51.Google Scholar
[7]Erdős, P., Frankl, P. and Rödl, V. (1986) The asymptotic number of graphs not containing a fixed subgraph and a problem for hypergraphs having no exponent. Graphs Combin. 2 113–121.CrossRefGoogle Scholar
[8]Erdős, P., Kleitman, D. J. and Rothschild, B. L. (1976) Asymptotic enumeration of Kn-free graphs. In Colloquio Internazionale sulle Teorie Combinatorie (Rome 1973), Vol. II, Accad. Naz. Lincei, Rome, pp. 19–27.Google Scholar
[9]Frankl, P. and Rödl, V. (1992) The uniformity lemma for hypergraphs. Graphs Combin. 8 309–312.CrossRefGoogle Scholar
[10]Füredi, Z. and Simonovits, M. (2005) Triple systems not containing a Fano configuration. Combin. Probab. Comput. 14 467–484.CrossRefGoogle Scholar
[11]Keevash, P. and Sudakov, B. (2005) The Turán number of the Fano plane. Combinatorica 25 561–574.CrossRefGoogle Scholar
[12]Kohayakawa, Y., Nagle, B., Rödl, V. and Schacht, M. Weak hypergraph regularity and linear hypergraphs. J. Combin. Theory, Sec. B, to appear.Google Scholar
[13]Kolaitis, P. G., Prömel, H. J. and Rothschild, B. L. (1985) Asymptotic enumeration and a 0–1 law for m-clique free graphs. Bull. Amer. Math. Soc. (N.S.) 13 160–162.CrossRefGoogle Scholar
[14]Kolaitis, P. G., Prömel, H. J. and Rothschild, B. L. (1987) K l+1-free graphs: Asymptotic structure and a 0–1 law. Trans. Amer. Math. Soc. 303 637–671.Google Scholar
[15]Nagle, B. and Rödl, V. (2001) The asymptotic number of triple systems not containing a fixed one. Discrete Math. 235 271–290.CrossRefGoogle Scholar
[16]Nagle, B., Rödl, V. and Schacht, M. (2006) Extremal hypergraph problems and the regularity method. In Topics in Discrete Mathematics, Algorithms Combin., Vol. 26, Springer, Berlin, pp. 247–278.CrossRefGoogle Scholar
[17]Person, Y. and Schacht, M. (2009) Almost all hypergraphs without Fano planes are bipartite. In Proc. Twentieth Annual ACM–SIAM Symposium on Discrete Algorithms (Mathieu, C., ed.), ACM, pp. 217–226.Google Scholar
[18]Steger, A. (1990) Die Kleitman–Rothschild Methode. PhD thesis, Forschungsinstitut für Diskrete Mathematik, Rheinische Friedrichs–Wilhelms–Universität Bonn.Google Scholar
[19]Szemerédi, E. (1978) Regular partitions of graphs. In Problèmes Combinatoires et Théorie des Graphes (Colloq. Internat. CNRS, Orsay 1976), CNRS, Paris, pp. 399–401.Google Scholar
[20]Yuster, R. (1996) The number of edge colorings with no monochromatic triangle. J. Graph Theory 21 441–452.3.0.CO;2-I>CrossRefGoogle Scholar