Article contents
Irregular subgraphs
Published online by Cambridge University Press: 23 September 2022
Abstract
We suggest two related conjectures dealing with the existence of spanning irregular subgraphs of graphs. The first asserts that any
 $d$
-regular graph on
$d$
-regular graph on
 $n$
vertices contains a spanning subgraph in which the number of vertices of each degree between
$n$
vertices contains a spanning subgraph in which the number of vertices of each degree between
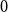 $0$
and
$0$
and
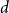 $d$
deviates from
$d$
deviates from
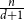 $\frac{n}{d+1}$
by at most
$\frac{n}{d+1}$
by at most
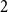 $2$
. The second is that every graph on
$2$
. The second is that every graph on
 $n$
vertices with minimum degree
$n$
vertices with minimum degree
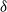 $\delta$
contains a spanning subgraph in which the number of vertices of each degree does not exceed
$\delta$
contains a spanning subgraph in which the number of vertices of each degree does not exceed
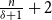 $\frac{n}{\delta +1}+2$
. Both conjectures remain open, but we prove several asymptotic relaxations for graphs with a large number of vertices
$\frac{n}{\delta +1}+2$
. Both conjectures remain open, but we prove several asymptotic relaxations for graphs with a large number of vertices
 $n$
. In particular we show that if
$n$
. In particular we show that if
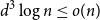 $d^3 \log n \leq o(n)$
then every
$d^3 \log n \leq o(n)$
then every
 $d$
-regular graph with
$d$
-regular graph with
 $n$
vertices contains a spanning subgraph in which the number of vertices of each degree between
$n$
vertices contains a spanning subgraph in which the number of vertices of each degree between
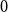 $0$
and
$0$
and
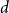 $d$
is
$d$
is
 $(1+o(1))\frac{n}{d+1}$
. We also prove that any graph with
$(1+o(1))\frac{n}{d+1}$
. We also prove that any graph with
 $n$
vertices and minimum degree
$n$
vertices and minimum degree
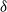 $\delta$
contains a spanning subgraph in which no degree is repeated more than
$\delta$
contains a spanning subgraph in which no degree is repeated more than
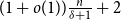 $(1+o(1))\frac{n}{\delta +1}+2$
times.
$(1+o(1))\frac{n}{\delta +1}+2$
times.
Keywords
- Type
- Paper
- Information
- Copyright
- © The Author(s), 2022. Published by Cambridge University Press
Footnotes
Research supported in part by NSF grant DMS-2154082 and BSF grant 2018267.
Research supported by NSF Award DMS-1953958.
References
- 4
- Cited by



