Hostname: page-component-cd9895bd7-hc48f Total loading time: 0 Render date: 2024-12-24T13:38:32.164Z Has data issue: false hasContentIssue false
  • English
  • Français

Weighted Norm Inequalities for a Maximal Operator in Some Subspace of Amalgams

Published online by Cambridge University Press:  20 November 2018

Justin Feuto ,
Ibrahim Fofana  and
Konin Koua
Justin Feuto
Affiliation:
UFR de Mathematiques et Informatique, Université de Cocody, Abidjan, République de Côte d’Ivoire e-mail: [email protected] e-mail: fofana ib math [email protected] e-mail: [email protected]
Ibrahim Fofana
Affiliation:
UFR de Mathematiques et Informatique, Université de Cocody, Abidjan, République de Côte d’Ivoire e-mail: [email protected] e-mail: fofana ib math [email protected] e-mail: [email protected]
Konin Koua
Affiliation:
UFR de Mathematiques et Informatique, Université de Cocody, Abidjan, République de Côte d’Ivoire e-mail: [email protected] e-mail: fofana ib math [email protected] e-mail: [email protected]
Rights & Permissions [Opens in a new window]

Abstract

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

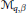We give weighted norm inequalities for the maximal fractional operator ${{\mathcal{M}}_{q}},\beta $ of Hardy–Littlewood and the fractional integral 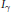${{I}_{\gamma }}$. These inequalities are established between 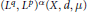${{\left( {{L}^{q}},\,{{L}^{p}} \right)}^{\alpha }}\left( X,\,d,\,\mu \right)$ spaces (which are superspaces of Lebesgue spaces 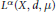${{L}^{\alpha }}\left( X,\,d,\,\mu \right)$ and subspaces of amalgams 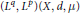$\left( {{L}^{q}},\,{{L}^{p}} \right)\left( X,d,\mu \right)$) and in the setting of space of homogeneous type 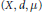$\left( X,d,\mu \right)$. The conditions on the weights are stated in terms of Orlicz norm.

Keywords

42B3542B2042B25fractional maximal operatorfractional integralspace of homogeneous type
Type
Research Article
Information
Canadian Mathematical Bulletin , Volume 53 , Issue 2 , 01 June 2010 , pp. 263 - 277
Copyright
Copyright © Canadian Mathematical Society 2010

References

[1] Bernardis, A. and Salinas, O., Two-weight norm inequalities for the fractional maximal operator on spaces of homogeneous type. Studia Math. 108(1994), no. 3, 201–207.Google Scholar
[2] Calderón, A. P., Inequalities for the maximal function relative to a metric. Studia Math. 57(1976), no. 3, 297–306.Google Scholar
[3] Chiarenza, F. and Frasca, M., Morrey spaces and Hardy-Littlewood maximal function Rend. Math. Appl. 7(1987), no. 3–4, 273–279.Google Scholar
[4] Eridani, A., Kokilashvili, V., and Meskhi, A., Morrey spaces and fractional integral operators. Expo. Math. 27(2009), no. 3, 227–239.Google Scholar
[5] Feuto, J., Fofana, I. and Koua, K., Integrable fractional mean functions on spaces of homogeneous type. Afr. Diaspora J. Math. 9(2010), no. 1, 8–30.Google Scholar
[6] Fofana, I., Étude d’une classe d’espaces de fonctions contenant les espaces de Lorentz. Afrika Mat 1(1988), 29–50.Google Scholar
[7] Fofana, I., Continuité de l’intégrale fractionnaire et espace (Lq, ℓp ) α . C. R. Acad. Sci. Paris Sér. I Math. 18(1989), 525–527.Google Scholar
[8] Fofana, I., Espaces (Lq, ℓp ) α et continuité de l’opérateur maximal fractionnaire de Hardy-Littelwood. Afrika Mat. 12(2001), 23–37.Google Scholar
[9] Founier, J. J. F. and Stewart, J., Amalgams of Lp and lq . Bull. Amer. Math. Soc. 13(1985), no. 1, 1–21.Google Scholar
[10] Han, Y., Müller, D., and Yang, D., A theory of Besov and Triebel-Lizorkin spaces on metric measure space modeled on Carnot-Carathéodory spaces. Abstr. Appl. Anal. (2008) Art. ID 893409, 252 pp.Google Scholar
[11] Kpata, A., Fofana, I., and Koua, K., Necessary condition for measures which are (Lq, Lp ) multipliers. Ann. Math. Blaise Pascal 16(2009), no. 2, 423–437.Google Scholar
[12] Macías, R. and Segovia, C., Lipschitz functions on spaces of homogeneous type. Adv. in Math. 33(1979), no. 3, 257–270. doi:10.1016/0001-8708(79)90012-4Google Scholar
[13] Mascré, D., Inégalités à poids pour l’opérateur de Hardy-Littlewood-Sobolev dans les espaces métriques mesurés à deux demi-dimensions. Colloq. Math. 105(2006), no. 1, 77–104. doi:10.4064/cm105-1-9Google Scholar
[14] Muckenhoupt, B. and Wheeden, R., Weighted norm inequalities for fractional integrals. Trans. Amer. Math. Soc. 192(1974), 261–274. doi:10.2307/1996833Google Scholar
[15] Nakai, E., Hardy-Littlewood maximal operator, singular integral operators and the Riez potentials on generalized Morrey spaces. Math. Nachr. 166(1994), 95–103. doi:10.1002/mana.19941660108Google Scholar
[16] Pérez, C. and Wheeden, R. L., Uncertainty principle estimates for vector fields. J. Funct. Anal. 181(2001), no. 1, 146–188. doi:10.1006/jfan.2000.3711Google Scholar
[17] Pérez, C. and Wheeden, R. L., Potential operators, maximal functions, and generalization of A ∞ . Potential Anal. 19(2003), no. 1, 1–33. doi:10.1023/A:1022449810008Google Scholar
[18] Rao, M. and Ren, Z., Theory of Orlicz Spaces. Monographs and Textbooks in Pure and Applied Mathematics 146. Marcel Dekker, New York, 1991.Google Scholar
[19] Sawyer, E. T. and Wheeden, R. L., Weighted inequalities for fractional integrals on Euclidean and homogeneous spaces. Amer. J. Math. 114(1992), no. 4, 813–874. doi:10.2307/2374799Google Scholar
[20] Varopoulos, N. T., Analysis on Lie groups. J. Funct. Anal. 76(1988), no. 2, 346–410. doi:10.1016/0022-1236(88)90041-9Google Scholar
[21] Jie, P. Wen, Fractional integrals on spaces of homogeneous type Approx. Theory Appl. 8(1992), no. 1, 1–15.Google Scholar
[22] Wiener, N., On the representation of functions by trigonometrical integrals. Math. Z. 24(1926), no. 1, 575–616. doi:10.1007/BF01216799Google Scholar