No CrossRef data available.
Published online by Cambridge University Press: 04 October 2021
We show that any weakly separated Bessel system of model spaces in the Hardy space on the unit disc is a Riesz system and we highlight some applications to interpolating sequences of matrices. This will be done without using the recent solution of the Feichtinger conjecture, whose natural generalization to multidimensional model subspaces of 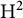 ${\mathrm {H}}^2$
turns out to be false.
${\mathrm {H}}^2$
turns out to be false.
The author was partially supported by National Science Foundation Grant DMS 1565243.