Hostname: page-component-78c5997874-ndw9j Total loading time: 0 Render date: 2024-11-03T02:24:56.297Z Has data issue: false hasContentIssue false
  • English
  • Français

Weak Factorizations of the Hardy Space H1(ℝn) in Terms of Multilinear Riesz Transforms

Published online by Cambridge University Press:  20 November 2018

Ji Li  and
Brett D. Wick
Ji Li
Affiliation:
Department of Mathematics, Macquarie University, NSW, 2109, Australia. e-mail: [email protected]
Brett D. Wick
Affiliation:
Department of Mathematics, Washington University–St. Louis, St. Louis, MO 63130-4899 USA. e-mail: [email protected]
Rights & Permissions [Opens in a new window]

Abstract

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

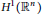This paper provides a constructive proof of the weak factorization of the classical Hardy space ${{H}^{1}}({{\mathbb{R}}^{n}})$ in terms of multilinear Riesz transforms. As a direct application, we obtain a new proof of the characterization of $BMO({{\mathbb{R}}^{n}})$ (the dual of 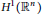${{H}^{1}}({{\mathbb{R}}^{n}})$) via commutators of the multilinear Riesz transforms.

Keywords

42B3542B20Hardy spaceBMO spacemultilinear Riesz transformweak factorization
Type
Research Article
Information
Canadian Mathematical Bulletin , Volume 60 , Issue 3 , 01 September 2017 , pp. 571 - 585
Copyright
Copyright © Canadian Mathematical Society 2017

References

[1] Chaffee, L., Characterizations of bounded mean oscillation through commutators of bilinear singular integral operators. Proc. Roy. Soc. Edinburgh Sect. A 146(2016), no. 6,1159-1166. http://dx.doi.Org/1 0.101 7/S030821051 5000888 Google Scholar
[2] Coifman, R. R., Rochberg, R., and Weiss, G., Factorization theorems for Hardy spaces in several variables. Ann. of Math. (2) 103(1976), no. 3, 611–635. http://dx.doi.Org/10.2307/1970954 Google Scholar
[3] Duong, X. T., Li, J., Wick, B. D., and Yang, D., Factorization for Hardy spaces and characterization for BMO spaces via commutators in the Bessel setting. Indiana Math. J., to appear. arxiv:1509.00079 http://dx.doi.Org/10.1007/s12220-011-9268-y Google Scholar
[4] Grafakos, L., Classical Fourier analysis. Second ed., Graduate Texts in Mathematics, 249, Springer, New York, 2008. Google Scholar
[5] Grafakos, L. and Torres, R. H., Multilinear Calderon-Zygmund theory. Adv. Math. 165(2002), no. 1, 124–164. http://dx.doi.Org/10.1006/aima.2001.2028 Google Scholar
[6] Janson, S., Mean oscillation and commutators of singular integral operators. Ark. Mat. 16(1978), no. 2, 263–270. http://dx.doi.Org/10.1007/BF02386000 Google Scholar
[7] Lerner, A. K., Ombrosi, S., Perez, C., Torres, R. H., and R. Trujillo-Gonzalez, New maximal functions and multiple weights for the multilinear Calderon-Zygmund theory. Adv. Math. 220(2009), no. 4, 1222–1264. http://dx.doi.Org/10.1016/j.aim.2008.10.014 Google Scholar
[8] Li, J., and Wick, B. D., Characterizations ofH1^ (R“) and BMOAN (R“) via weak factorizations and commutators. arxiv:1505.04375 Google Scholar
[9] Perez, C., Pradolini, G., Torres, R. H., and Trujillo-Gonzalez, R., End-point estimates for iterated commutators of multilinear singular integrals. Bull. Lond. Math. Soc. 46(2014), no. 1, 26–42. http://dx.doi.Org/1 0.1112/blms/bdtO65 Google Scholar
[10] Perez, C. and Torres, R. H., Sharp maximal function estimates for multilinear singular integrals. In: Harmonic analysis at Mount Holyoke (South Hadley, MA, 2001), Contemp. Math., 320, American Mathematical Society, Providence, RI, 2003, pp. 323–331. http://dx.doi.Org/10.1090/conm/320/05615 Google Scholar
[11] Tang, L., Weighted estimates for vector-valued commutators of multilinear operators. Proc. Roy. Soc. Edinburgh Sect. A 138(2008), no. 4, 897–922. http://dx.doi.Org/1 0.101 7/S0308210504000976 Google Scholar
[12] Uchiyama, A., The factorization ofH? on the space of homogeneous type. Pacific J. Math. 92(1981), no. 2,453-468. http://dx.doi.Org/10.2140/pjm.1981.92.453 Google Scholar