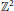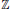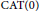Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by Crossref.
Brady, Noel
and
Soroko, Ignat
2019.
Dehn functions of subgroups of right-angled Artin groups.
Geometriae Dedicata,
Vol. 200,
Issue. 1,
p.
197.
Button, J. O.
2019.
Tubular groups, 1-relator groups and nonpositive curvature.
International Journal of Algebra and Computation,
Vol. 29,
Issue. 08,
p.
1367.
Hoda, Nima
Wise, Daniel T.
and
Woodhouse, Daniel J.
2020.
Residually finite tubular groups.
Proceedings of the Royal Society of Edinburgh: Section A Mathematics,
Vol. 150,
Issue. 6,
p.
2937.
Bregman, Corey
and
Clay, Matt
2021.
Minimal volume entropy of free-by-cyclic groups and 2-dimensional right-angled Artin groups.
Mathematische Annalen,
Vol. 381,
Issue. 3-4,
p.
1253.
Dudkin, F. A.
and
Usikov, A. V.
2024.
Residual π-Finiteness of Tubular Groups.
Algebra and Logic,
Vol. 63,
Issue. 1,
p.
28.