Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by Crossref.
Lau, A. T.-M.
1990.
The Analytical and Topological Theory of Semigroups.
p.
313.


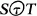 a semidirect product. Conditions are determined under which topological left amenability of S and T implies that of
a semidirect product. Conditions are determined under which topological left amenability of S and T implies that of 