Hostname: page-component-cd9895bd7-8ctnn Total loading time: 0 Render date: 2024-12-24T18:17:17.556Z Has data issue: false hasContentIssue false
  • English
  • Français

The Time Change Method and SDEs with Nonnegative Drift

Published online by Cambridge University Press:  20 November 2018

V. P. Kurenok
V. P. Kurenok*
Affiliation:
Department of Natural and Applied Sciences, University of Wisconsin-Green Bay, 2420 Nicolet Drive, Green Bay, WI 54311-7001, USA e-mail: [email protected]
Rights & Permissions [Opens in a new window]

Abstract

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

Using the time change method we show how to construct a solution to the stochastic equation 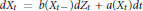$d{{X}_{t}}\,=\,b({{X}_{t}}\_)d{{Z}_{t}}\,+\,a({{X}_{t}})dt$ with a nonnegative drift a provided there exists a solution to the auxililary equation 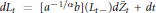$d{{L}_{t}}=[{{a}^{-1/\alpha }}b]({{L}_{t}}\_)d\overline{{{Z}_{t}}}+dt$ where 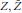$Z,\,\overline{Z}$ are two symmetric stable processes of the same index $\alpha \,\in \,(0,\,2]$. This approach allows us to prove the existence of solutions for both stochastic equations for the values 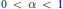$0\,<\,\alpha \,<\,1$ and only measurable coefficients $a$ and 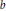$b$ satisfying some conditions of boundedness. The existence proof for the auxililary equation uses the method of integral estimates in the sense of Krylov.

Keywords

60H1060J6060J6560G44One-dimensional SDEssymmetric stable processesnonnegative drifttime changeintegral estimatesweak convergence
Type
Research Article
Information
Canadian Mathematical Bulletin , Volume 53 , Issue 3 , 01 September 2010 , pp. 503 - 515
Copyright
Copyright © Canadian Mathematical Society 2010

References

[1] Aldous, D., Stopping times and tightness. Ann. Prob. 6(1978), no. 2, 335–340. doi:10.1214/aop/1176995579Google Scholar
[2] Carr, P., Geman, H., Madan, D., and Yor, M., Stochastic volatility for Lévy processes. Math. Finance 13(2003), no. 3, 345–382. doi:10.1111/1467-9965.00020Google Scholar
[3] Carr, P. and Wu, L., Time-changed Lévy processes and option pricing. J. Financial Economics, 71(2004), no. 1, 113–141.Google Scholar
[4] Dellacherie, C., Meyer, P.-A., Probabilités et potentiels. Hermann, Paris, 1980.Google Scholar
[5] Engelbert, H.-J. and Kurenok, V. P., On one-dimensional stochastic equations driven by symmetric stable processes. In: Stochastic Processes and Related Topics, Taylor and Francis, London, 2002, pp. 81–109.Google Scholar
[6] Engelbert, H. J. and Schmidt, W.. On solutions of one-dimensional stochastic differential equations without drift. Z. Wahrsch. Verw. Gebiete 68(1985), no. 3, 287–314. doi:10.1007/BF00532642Google Scholar
[7] Engelbert, H. J. and Schmidt, W., On one-dimensional stochastic differential equations with generalized drift. In: Lecture Notes in Control and Inform Sci. 69, Springer, Berlin, 1985, pp. 143–155.Google Scholar
[8] Engelbert, H. J. and Schmidt, W., Strong Markov continuous local martingales and solutions of one-dimensional stochastic differential equations III. Math. Nachr. 151(1991), 149–197. doi:10.1002/mana.19911510111Google Scholar
[9] Ikeda, N. and Watanabe, S., Stochastic differential equations and diffusion processes. Second ed., North-Holland Publishing Co., Amsterdam, 1989.Google Scholar
[10] Jacod, J., Calcul stochastique et problèmes de martingales. Lecture Notes in Mathematics 714, Springer, Berlin, 1979.Google Scholar
[11] Kurenok, V. P., A note on L 2 -estimates for stable integrals with drift. Trans. Amer. Math. Soc. 360(2008), no. 2, 925–938. doi:10.1090/S0002-9947-07-04234-1Google Scholar
[12] Kurenok, V. P.. Stochastic equations driven by a Cauchy process. In: Markov Processes and Related Topics: A Festschrift for Thomas G. Kurtz, Institute of Mathematical Statistics Collections 4, Institute of Mathematical Statistics, Beachwood, Ohio, 2008, pp. 99–106.Google Scholar
[13] Krylov, N. V., Controlled diffusion processes. In: Applications of Mathematics 14, Springer, New York, 1980.Google Scholar
[14] Portenko, N. I., Some perturbations of drift-type for symmetric stable processes. Random Oper. Stochastic Equations 2, no. 3, 211–224. doi:10.1515/rose.1994.2.3.211Google Scholar
[15] Pragarauskas, G. and Zanzotto, P. A., On one-dimensional stochastic differential equations driven by stable processes. Liet. Mat. Rink., 40, 1–24.Google Scholar
[16] Revuz, D. and Yor, M., Continuous martingales and Brownian motion. Third ed., Fundamental Principles of Mathematical Sciences 293, Springer, Berlin, 1999.Google Scholar
[17] Rosiński, J. and Woyczyński, W. A., On Itô stochastic integration with respect to p-stable motion: inner clock, integrability of sample paths, double and multiple integrals. Ann. Probab. 14, no. 1, 271–286. doi:10.1214/aop/1176992627Google Scholar
[18] Tanaka, H., Tsuchiya, M., and S.Watanabe, Perturbation of drift-type for Lévy processes. J. Math. Kyoto Univ. 14(1974), 73–92.Google Scholar
[19] Zanzotto, P. A., On solutions of one-dimensional stochastic differential equations driven by stable Lévy motion. Stochastic Process. Appl. 68(1997), no. 2, 209–228. doi:10.1016/S0304-4149(97)00030-6Google Scholar
[20] Zanzotto, P. A., On stochastic differential equations driven by a Cauchy process and the other stable Lévy motions. Ann. Probab. 30(2002), no. 2, 802–825. doi:10.1214/aop/1023481008Google Scholar