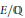Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by Crossref.
Anni, Samuele
and
Siksek, Samir
2015.
On Serre’s uniformity conjecture for semistable elliptic curves over totally real fields.
Mathematische Zeitschrift,
Vol. 281,
Issue. 1-2,
p.
193.