Published online by Cambridge University Press: 13 December 2021
Bezdek and Kiss showed that existence of origin-symmetric coverings of unit sphere in
 ${\mathbb {E}}^n$
by at most
${\mathbb {E}}^n$
by at most
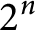 $2^n$
congruent spherical caps with radius not exceeding
$2^n$
congruent spherical caps with radius not exceeding
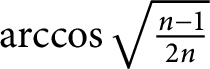 $\arccos \sqrt {\frac {n-1}{2n}}$
implies the X-ray conjecture and the illumination conjecture for convex bodies of constant width in
$\arccos \sqrt {\frac {n-1}{2n}}$
implies the X-ray conjecture and the illumination conjecture for convex bodies of constant width in
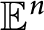 ${\mathbb {E}}^n$
, and constructed such coverings for
${\mathbb {E}}^n$
, and constructed such coverings for
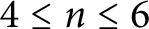 $4\le n\le 6$
. Here, we give such constructions with fewer than
$4\le n\le 6$
. Here, we give such constructions with fewer than
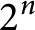 $2^n$
caps for
$2^n$
caps for
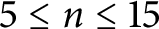 $5\le n\le 15$
.
$5\le n\le 15$
.
For the illumination number of any convex body of constant width in
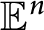 ${\mathbb {E}}^n$
, Schramm proved an upper estimate with exponential growth of order
${\mathbb {E}}^n$
, Schramm proved an upper estimate with exponential growth of order
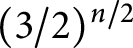 $(3/2)^{n/2}$
. In particular, that estimate is less than
$(3/2)^{n/2}$
. In particular, that estimate is less than
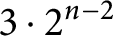 $3\cdot 2^{n-2}$
for
$3\cdot 2^{n-2}$
for
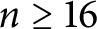 $n\ge 16$
, confirming the abovementioned conjectures for the class of convex bodies of constant width. Thus, our result settles the outstanding cases
$n\ge 16$
, confirming the abovementioned conjectures for the class of convex bodies of constant width. Thus, our result settles the outstanding cases
 $7\le n\le 15$
.
$7\le n\le 15$
.
We also show how to calculate the covering radius of a given discrete point set on the sphere efficiently on a computer.
The first author was supported in part by Grant 275113 of the Research Council of Norway. The second author was supported by NSERC of Canada Discovery Grant RGPIN-2020-05357.