Hostname: page-component-cd9895bd7-lnqnp Total loading time: 0 Render date: 2024-12-23T01:23:58.186Z Has data issue: false hasContentIssue false
  • English
  • Français

Spaces of Quasi-Measures

Published online by Cambridge University Press:  20 November 2018

D. J. Grubb  and
Tim LaBerge
D. J. Grubb
Affiliation:
Department of Mathematical Sciences Northern Illinois University DeKalb, IL 60115 USA, email: [email protected] Website: http://www.math.niu.edu/∼grubb/
Tim LaBerge
Affiliation:
Department of Mathematics Centenary College Shreveport, LA 71134 USA, email: [email protected] Website: http://www.centenary.edu/∼laberget/
Rights & Permissions [Opens in a new window]

Abstract

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

We give a direct proof that the space of Baire quasi-measures on a completely regular space (or the space of Borel quasi-measures on a normal space) is compact Hausdorff. We show that it is possible for the space of Borel quasi-measures on a non-normal space to be non-compact. This result also provides an example of a Baire quasi-measure that has no extension to a Borel quasi-measure. Finally, we give a concise proof of the Wheeler-Shakmatov theorem, which states that if 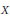$X$ is normal and 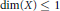$\dim\left( X \right)\,\le \,1$, then every quasi-measure on 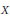$X$ extends to a measure.

Keywords

28C15
Type
Research Article
Information
Canadian Mathematical Bulletin , Volume 42 , Issue 3 , 01 September 1999 , pp. 291 - 297
Copyright
Copyright © Canadian Mathematical Society 1999

References

[A1] Aarnes, J., Quasi-states and quasi-measures. Adv.Math. 86 (1991), 41–67.Google Scholar
[A2] Aarnes, J., Pure quasi-states and extremal quasi-measures. Math. Ann. 295 (1993), 575–588.Google Scholar
[A3] Aarnes, J., Construction of non-subadditive measures and discretization of Borel measures. Fund. Math. 147 (1995), 213–237.Google Scholar
[BS] Bachman, G. and Sultan, A., On regular extensions of measures. Pacific J. Math. 86 (1980), 389–395.Google Scholar
[B1] Boardman, J., Quasi-measures on completely regular spaces. Doctoral dissertation, Northern Illinois University, 1994.Google Scholar
[B2] Boardman, J., Quasi-measures on completely regular spaces. Rocky Mountain J. Math. (2) 27 (1997), 447–470.Google Scholar
[F] Fremlin, D., On quasi-measures. Preprint.Google Scholar
[S] Shakmatov, D., Linearity of quasi-states on commutative C*-algebras of stable rank 1. Preprint.Google Scholar
[T] Topsoe, F., Compactness in spaces of measures. Studia Math. 36 (1970), 195–212.Google Scholar
[W] Wheeler, R. F., Quasi-measures and dimension theory. Topology Appl. 66 (1995), 75–92.Google Scholar