Hostname: page-component-cd9895bd7-dk4vv Total loading time: 0 Render date: 2024-12-25T17:55:29.610Z Has data issue: false hasContentIssue false
  • English
  • Français

Small Sets of k-th Powers

Published online by Cambridge University Press:  20 November 2018

Ping Ding  and
A. R. Freedman
Ping Ding
Affiliation:
Department of Mathematics and Statistics, Simon Fraser University, Burnaby, British Columbia, V5A 1S6
A. R. Freedman
Affiliation:
Department of Mathematics and Statistics, Simon Fraser University, Burnaby, British Columbia, V5A 1S6
Rights & Permissions [Opens in a new window]

Abstract

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

Let k ≥ 2 and q = g(k) — G(k), where g(k) is the smallest possible value of r such that every natural number is the sum of at most r k-th powers and G(k) is the minimal value of r such that every sufficiently large integer is the sum of r k-th powers. For each positive integer r ≥ q, let 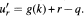Then for every ε > 0 and N ≥ N(r, ε), we construct a set A of k-th powers such that |A| ≤ (r(2 + ε)r + l)N1/(k+r) and every nonnegative integer n ≤ N is the sum of 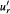k-th powers in A. Some related results are also obtained.

Keywords

11P05
Type
Research Article
Information
Canadian Mathematical Bulletin , Volume 37 , Issue 2 , 01 June 1994 , pp. 168 - 173
Copyright
Copyright © Canadian Mathematical Society 1994

References

1. Choi, S. L. G., Erdôs, P. and Nathanson, M. B., Lagrange's theorem with N1/3 squares, Proc. Amer. Math. Soc. (2) 79(1980), 203–205.Google Scholar
2. Landau, E., Handbuch derLehre von der Verteilung der Primzahlen, Teubner, Leipzig, 1909.Google Scholar
3. Linnik, Yu. V., An elementary solution of a problem of Waring by Schnirelmann's method, Mat. Sb. (54) 12(1943), 225–230.Google Scholar
4. Nathanson, M. B., Waring's problem for sets of density zero, Analytic Number Theory, Lecture Notes in Math., Springer-Verlag, Berlin 899, 301–310.Google Scholar
5. Ribenboim, P., The book of prime number records, Second edition, Springer-Verlag, New York, 1989.Google Scholar
6. Vaughan, R. C., A new iterative method in Waring s problem, Acta Math. 162(1989), 1–71.Google Scholar
7. Vaughan, R. C. and Wooley, T. D., On Waring's problem: some refinements, Proc. London Math. Soc. (3) 63(1991), 35–68.Google Scholar
8. Wieferich, A., Beweis des Statzes, dass sich eine jede ganze Zahl als Summe von hochsten neun positiven Kuben darstellen lasst, Math. Ann. 66(1909), 95–101.Google Scholar