Published online by Cambridge University Press: 20 November 2018
Let  $G$ be a metrizable compact group,
$G$ be a metrizable compact group,  $A$ a separable
$A$ a separable 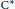 ${{\text{C}}^{*}}$-algebra, and
${{\text{C}}^{*}}$-algebra, and  $\alpha :G\,\to \,\text{Aut}\left( A \right)$ a strongly continuous action. Provided that
$\alpha :G\,\to \,\text{Aut}\left( A \right)$ a strongly continuous action. Provided that  $\alpha $ satisfies the continuous Rokhlin property, we show that the property of satisfying the
$\alpha $ satisfies the continuous Rokhlin property, we show that the property of satisfying the 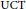 $\text{UCT}$ in
$\text{UCT}$ in  $E$-theory passes from
$E$-theory passes from  $A$ to the crossed product
$A$ to the crossed product 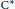 ${{\text{C}}^{*}}$-algebra
${{\text{C}}^{*}}$-algebra 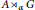 $\mathcal{A}{{\rtimes }_{\alpha }}\,G$ and the fixed point algebra
$\mathcal{A}{{\rtimes }_{\alpha }}\,G$ and the fixed point algebra 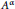 ${{A}^{\alpha }}$. This extends a similar result by Gardella for
${{A}^{\alpha }}$. This extends a similar result by Gardella for 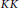 $KK$-theory in the case of unital
$KK$-theory in the case of unital 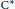 ${{\text{C}}^{*}}$-algebras but with a shorter and less technical proof. For circle actions on separable unital
${{\text{C}}^{*}}$-algebras but with a shorter and less technical proof. For circle actions on separable unital 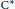 ${{\text{C}}^{*}}$-algebras with the continuous Rokhlin property, we establish a connection between the
${{\text{C}}^{*}}$-algebras with the continuous Rokhlin property, we establish a connection between the  $E$-theory equivalence class of
$E$-theory equivalence class of  $A$ and that of its fixed point algebra
$A$ and that of its fixed point algebra 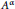 ${{A}^{\alpha }}$.
${{A}^{\alpha }}$.