Let $A\,:=\,-\,\left( \nabla \,-\,i\overrightarrow{a} \right)\,.\,\left( \nabla \,-\,i\overrightarrow{a} \right)\,+\,V$ be a magnetic Schrödinger operator on 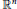${{\mathbb{R}}^{n}}$, where

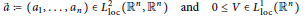$$\vec{a}:=\left( {{a}_{1}},...,{{a}_{n}} \right)\in L_{\text{loc}}^{2}\left( {{\mathbb{R}}^{n}},{{\mathbb{R}}^{n}} \right)\operatorname{and}0\le V\in L_{\text{loc}}^{1}\left( {{\mathbb{R}}^{n}} \right)$$

satisfy some reverse Hölder conditions. Let 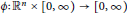$\phi :\,{{\mathbb{R}}^{n}}\,\times \,[0,\,\infty )\,\to \,[0,\,\infty )$ be such that $\phi \left( x,\,. \right)$ for any given 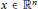$x\,\in \,{{\mathbb{R}}^{n}}$ is an Orlicz function, 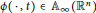$\phi \left( ^{.}\,,\,t \right)\,\in \,{{\mathbb{A}}_{\infty }}\left( {{\mathbb{R}}^{n}} \right)$ for all $t\,\in \,\left( 0,\,\infty \right)$ (the class of uniformly Muckenhoupt weights) and its uniformly critical upper type index 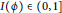$I\left( \phi \right)\,\in \,(0,\,1]$. In this article, the authors prove that second-order Riesz transforms 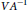$V{{A}^{-1}}$ and 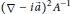${{\left( \nabla \,-\,i\overrightarrow{a} \right)}^{2}}{{A}^{-1}}$ are bounded from the Musielak–Orlicz–Hardy space 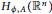${{H}_{\phi ,\,A}}\left( {{\mathbb{R}}^{n}} \right)$, associated with 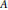$A$, to theMusielak–Orlicz space 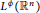${{L}^{\phi }}\left( {{\mathbb{R}}^{n}} \right)$. Moreover, we establish the boundedness of 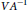$V{{A}^{-1}}$ on 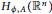${{H}_{\phi ,\,A}}\left( {{\mathbb{R}}^{n}} \right)$. As applications, some maximal inequalities associated with 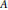$A$ in the scale of 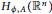${{H}_{\phi ,\,A}}\left( {{\mathbb{R}}^{n}} \right)$ are obtained.