Hostname: page-component-cd9895bd7-jn8rn Total loading time: 0 Render date: 2024-12-24T02:53:28.497Z Has data issue: false hasContentIssue false
  • English
  • Français

Schubert Calculus on a Grassmann Algebra

Published online by Cambridge University Press:  20 November 2018

Letterio Gatto  and
Taíse Santiago
Letterio Gatto
Affiliation:
Dipartimento di Matematica, Politecnico di Torino, C.so Duca degli Abruzzi, 24, 10129 Torino, Italy e-mail: [email protected]
Taíse Santiago
Affiliation:
Instituto de Matemática, Universidade Federal da Bahia, Av. Ademar Barros S/N, Ondina, Salvador-Bahia, 40170-110, (BA), Brazil e-mail: [email protected]
Rights & Permissions [Opens in a new window]

Abstract

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

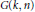The (classical, small quantum, equivariant) cohomology ring of the grassmannian $G\left( k,\,n \right)$ is generated by certain derivations operating on an exterior algebra of a free module of rank $n$ (Schubert calculus on a Grassmann algebra). Our main result gives, in a unified way, a presentation of all such cohomology rings in terms of generators and relations. Using results of Laksov and Thorup, it also provides a presentation of the universal factorization algebra of a monic polynomial of degree $n$ into the product of two monic polynomials, one of degree $k$.

Keywords

14N1514M15
Type
Research Article
Information
Canadian Mathematical Bulletin , Volume 52 , Issue 2 , 01 June 2009 , pp. 200 - 212
Copyright
Copyright © Canadian Mathematical Society 2009

References

[1] Bertram, A., Quantum Schubert calculus. Adv. Math. 128(1997), no. 2, 289–305.Google Scholar
[2] Fulton, W., Intersection Theory. Ergebnisse der Mathematik und ihrer Grenzgebiete 3, Springer-Verlag, Berlin, 1984.Google Scholar
[3] Fulton, W., Equivariant Intersection Theory. The Eilenberg Lectures at Columbia University, 2007. Notes by D. Anderson available at http://www.math.lsa.umich.edu/_dandersn/eilenberg.Google Scholar
[4] Gatto, L., Schubert calculus via Hasse–Schmidt derivations. Asian J. Math. 9(2005), no. 3, 315–321.Google Scholar
[5] Gatto, L., Schubert calculus: an algebraic introduction. IMPA Mathematical Publications, Rio de Janeiro, 2005.Google Scholar
[6] Gatto, L. and Santiago, T., Equivariant Schubert Calculus. http://calvino.polito.it/_gatto/public/lavori/preprints/equivariant.pdf. Google Scholar
[7] Knutson, A. and Tao, T. Puzzles and (equivariant) cohomology of Grassmannians. Duke Math. J. 119(2003), no. 2, 221–260.Google Scholar
[8] Laksov, D., The formalism of equivariant Schubert calculus. Adv. Math 217(2008), 1869–1888.Google Scholar
[9] Laksov, D. and Thorup, A., A determinantal formula for the exterior powers of the polynomial ring. Indiana Univ. Math. J. 56(2007), 825–846.Google Scholar
[10] Laksov, D. and Thorup, A., Schubert calculus on Grassmannians and exterior products. To appear in Indiana Univ. Math. J.Google Scholar
[11] Mihalcea, L. C., Giambelli formulae for the equivariant quantum cohomology of the Grassmannian. Trans. Am. Math. Soc. 360(2008), no. 5, 2285–2301.Google Scholar
[12] Santiago, T., Schubert calculus on a Grassmann algebra. Ph.D. Thesis, Politecnico di Torino, 2006.Google Scholar
[13] Siebert, B. and Tian, G., On quantum cohomology rings of Fano manifolds and a formula of Vafa and Intrilligator. Asian J. Math. 1(1997), no. 4, 679–695.Google Scholar
[14] Witten, E., The Verlinde algebra and the cohomology of the Grassmannian. In: Geometry, topology and physics, Conf. Proc. Lecture Notes Geom. Topology IV, International Press, Cambridge, MA, 1995, pp. 357–422.Google Scholar