Article contents
Relative Homotopy in Relational Structures
Published online by Cambridge University Press: 20 November 2018
Abstract
The homotopy groups of a finite partially ordered set (poset) can be described entirely in the context of posets, as shown in a paper by  $\text{B}$. Larose and
$\text{B}$. Larose and  $\text{C}$. Tardif. In this paper we describe the relative version of such a homotopy theory, for pairs
$\text{C}$. Tardif. In this paper we describe the relative version of such a homotopy theory, for pairs 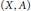 $\left( X,\,A \right)$ where
$\left( X,\,A \right)$ where 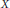 $X$ is a poset and
$X$ is a poset and  $A$ is a subposet of
$A$ is a subposet of 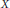 $X$. We also prove some theorems on the relevant version of the notion of weak homotopy equivalences for maps of pairs of such objects. We work in the category of reflexive binary relational structures which contains the posets as in the work of Larose and Tardif.
$X$. We also prove some theorems on the relevant version of the notion of weak homotopy equivalences for maps of pairs of such objects. We work in the category of reflexive binary relational structures which contains the posets as in the work of Larose and Tardif.
Keywords
- Type
- Research Article
- Information
- Copyright
- Copyright © Canadian Mathematical Society 2008
References
- 1
- Cited by


