Published online by Cambridge University Press: 20 November 2018
A 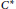 ${{C}^{*}}$-algebra Ahas the ideal property if any ideal
${{C}^{*}}$-algebra Ahas the ideal property if any ideal  $I$ of
$I$ of 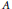 $A$ is generated as a closed two-sided ideal by the projections inside the ideal. Suppose that the limit
$A$ is generated as a closed two-sided ideal by the projections inside the ideal. Suppose that the limit 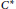 ${{C}^{*}}$-algebra
${{C}^{*}}$-algebra 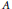 $A$ of inductive limit of direct sums of matrix algebras over spaces with uniformly bounded dimension has the ideal property. In this paper we will prove that
$A$ of inductive limit of direct sums of matrix algebras over spaces with uniformly bounded dimension has the ideal property. In this paper we will prove that 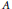 $A$ can be written as an inductive limit of certain very special subhomogeneous algebras, namely, direct sum of dimension-drop interval algebras and matrix algebras over 2-dimensional spaces with torsion
$A$ can be written as an inductive limit of certain very special subhomogeneous algebras, namely, direct sum of dimension-drop interval algebras and matrix algebras over 2-dimensional spaces with torsion 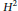 ${{H}^{2}}$ groups.
${{H}^{2}}$ groups.