Hostname: page-component-cd9895bd7-p9bg8 Total loading time: 0 Render date: 2024-12-23T16:26:37.395Z Has data issue: false hasContentIssue false
  • English
  • Français

The Oscillatory Hyper-Hilbert Transform Associated with Plane Curves

Published online by Cambridge University Press:  20 November 2018

Junfeng Li  and
Haixia Yu
Junfeng Li
Affiliation:
Laboratory of Math and Complex systems, Ministry of Education, School of Mathematical Sciences, Beijing Normal University, Beijing, 100875, China, e-mail : [email protected], [email protected]
Haixia Yu
Affiliation:
Laboratory of Math and Complex systems, Ministry of Education, School of Mathematical Sciences, Beijing Normal University, Beijing, 100875, China, e-mail : [email protected], [email protected]
Rights & Permissions [Opens in a new window]

Abstract

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

In this paper, the bounded properties of oscillatory hyper-Hilbert transformalong certain plane curves $\gamma \left( t \right)$,

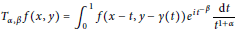$${{T}_{\alpha ,\beta }}f\left( x,\,y \right)\,=\,\int_{0}^{1}{f\left( x\,-\,t,\,y\,-\,\gamma \left( t \right) \right){{e}^{i{{t}^{-\beta }}}}\frac{\text{d}t}{{{t}^{1}}+\alpha }}$$

are studied. For general curves, these operators are bounded in ${{L}^{2}}\left( {{\mathbb{R}}^{2}} \right)$ if 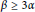$\beta \,\ge \,3\alpha $. Their boundedness in ${{L}^{p}}\left( {{\mathbb{R}}^{2}} \right)$ is also obtained, whenever 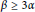$\beta \,\ge \,3\alpha $ and 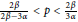$\frac{2\beta }{2\beta -3\alpha }\,<\,p\,<\,\frac{2\beta }{3\alpha }$.

Keywords

42B2042B35oscillatory hyper-Hilbert transformoscillatory integral
Type
Research Article
Information
Canadian Mathematical Bulletin , Volume 61 , Issue 4 , 01 December 2018 , pp. 802 - 811
Copyright
Copyright © Canadian Mathematical Society 2018

References

[1] Bez, N., LP-boundedness for the Hilbert transform and maximal operator along a class of nonconvex curves. Proc. Amer. Math. Soc. 135 (2007), no. 1, 151-161. http://dx.doi.org/10.1090/S0002-9939-06-08603-5Google Scholar
[2] Carbery, A., Christ, M., Vance, J., Wainger, S., and Watson, D., Operators associated to flat plane curves: LP estimates via dilation methods. Duke Math. J. 59 (1989), no. 3, 675-700. http://dx.doi.org/10.1215/S0012-7094-89-05930-9Google Scholar
[3] Carbery, A., Vance, J., Wainger, S., and Watson, D., The Hilbert transform and maximal function along flat curves, dilations, and differential equations. Amer. J. Math. 116 (1994), no. 5, 1203-1239. http://dx.doi.org/10.2307/2374944Google Scholar
[4] Carbery, A. and Ziesler, S., Hilbert transforms and maximal functions along rough flat curves. Rev. Mat. Iberoamericana 10 (1994), no. 2, 379-393. http://dx.doi.org/!0.4171/RM 1/156Google Scholar
[5] Carlsson, H., Christ, M., Cordoba, A., Duoandikoetxea, J., de Francia, J. L. Rubio, Vance, J., Wainger, S., and Weinberg, D., LP estimates for maximal functions and Hilbert transforms along flat convex curves in R2. Bull. Amer. Math. Soc. (N.S.) 14 (1986), no. 2, 263-267. http://dx.doi.org/10.1090/S0273-0979-1986-15433-9Google Scholar
[6] Chandarana, S., LP-bounds for hypersingular integral operators along curves. Pacific J. Math. 175 (1996), no. 2, 389-416. http://dx.doi.org/10.2140/pjm.1996.175.389Google Scholar
[7] Chen, J., Damtew, B., and Zhu, X., Oscillatory hyper Hilbert transforms along general curves. Front. Math. China 12 (2017), no. 2, 281-299. http://dx.doi.Org/10.1007/s11464-01 6-0574-3Google Scholar
[8] Chen, J., Fan, D., Wang, M., and Zhu, X., LP bounds for oscillatory hyper-Hilbert transform along curves. Proc. Amer. Math. Soc. 136 (2008), no. 9, 3145-3153. http://dx.doi.org/10.1090/S0002-9939-08-09325-8Google Scholar
[9] Chen, J., Fan, D., and Zhu, X., Sharp L2 boundedness of the oscillatory hyper-Hilbert transform along curves. Acta Math. Sin. (Engl. Ser.) 26 (2010), no. 4, 653-658. http://dx.doi.Org/10.1007/s10114-01 0-7396-0Google Scholar
[10] Christ, M., Hilbert transforms along curves. II. Aflat case. Duke Math. J. 52 (1985), no. 4, 887-894. http://dx.doi.org/10.1215/S0012-7094-85-05246-9Google Scholar
[11] Cordoba, A. and de Francia, J. L. Rubio, Estimates for Wainger's singular integrals along curves. Rev. Mat. Iberoamericana 2 (1986), no. 1-2,105-117. http://dx.doi.Org/10.4171/RMI/29Google Scholar
[12] Fefferman, C.. Inequality for strongly singular convolutions operators. Acta Math. 124 (1970), 9–36. http://dx.doi.org/10.1007/BF02394567Google Scholar
[13] Li, J. and Lu, S., Applications of the scale changing method to boundedness of certain commutators. Internat. J. Anal. Math. Sci. 1 (2004), 1–12.Google Scholar
[14] Nagel, A. and Wainger, S., Hilbert transforms associated with plane curves. Trans. Amer. Math. Soc. 223 (1976), 235–252. http://dx.doi.org/10.1090/S0002-9947-1976-0423010-8Google Scholar
[15] Stein, E. M., Harmonic analysis: real-variable methods, orthogonality, and oscillatory integrals. Princeton Mathematical Series, 43, Princeton University Press, Princeton, NJ, 1993.Google Scholar
[16] Stein, E. M. and Wainger, S., Problems in harmonic analysis related to curvature. Bull. Amer. Math. Soc. 84 (1978), no. 6,1239-1295. http://dx.doi.org/10.1090/S0002-9904-1978-14554-6Google Scholar
[17] Vance, J., Wainger, S., and Wright, J., The Hilbert transform and maximal function along nonconvex curves in the plane. Rev. Mat. Iberoamericana 10 (1994), no. 1, 93-121. http://dx.doi.Org/10.4171/RM1/146Google Scholar
[18] Wright, J., If estimates for operators associated to oscillating plane curves. Duke Math. J. 67 (1992), no. 1, 101-157. http://dx.doi.org/10.1215/S0012-7094-92-06705-6Google Scholar
[19] Zielinski, M., Highly oscillatory singular integrals along curves. Ph.D. Thesis, The University of Wisconsin - Madison, 1985.Google Scholar
[20] Ziesler, S., Lt'-boundedness of the Hilbert transform and maximal function associated to flat plane curves. Proc. Amer. Math. Soc. 122 (1994), no. 4, 1035-1043. http://dx.doi.Org/10.2307/2161171Google Scholar