Hostname: page-component-cd9895bd7-8ctnn Total loading time: 0 Render date: 2024-12-23T20:15:59.206Z Has data issue: false hasContentIssue false
  • English
  • Français

On the Theorem of the Primitive Element with Applications to the Representation Theory of Associative and Lie Algebras

Published online by Cambridge University Press:  20 November 2018

Leandro Cagliero  and
Fernando Szechtman
Leandro Cagliero
Affiliation:
CIEM-CONICET, FAMAF-Universidad Nacional de Córdoba, Cördoba, Argentina. e-mail: [email protected]
Fernando Szechtman
Affiliation:
Department of Mathematics and Statistics, University of Regina, Regina, SK e-mail: [email protected]
Rights & Permissions [Opens in a new window]

Abstract

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

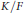We describe all finite dimensional uniserial representations of a commutative associative (resp. abelian Lie) algebra over a perfect (resp. sufficiently large perfect) field. In the Lie case the size of the field depends on the answer to following question, considered and solved in this paper. Let $K/F$ be a finite separable field extension and let 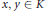$x,\,y\,\in \,K$. When is 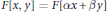$F\left[ x,\,y \right]\,=\,F\left[ \alpha x\,+\,\beta y \right]$ for some nonzero elements 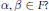$\alpha ,\,\beta \,\in \,F?$

Keywords

17B1013C0512F1012E20uniserial moduleLie algebraassociative algebraprimitive element
Type
Research Article
Information
Canadian Mathematical Bulletin , Volume 57 , Issue 4 , 01 December 2014 , pp. 735 - 748
Copyright
Copyright © Canadian Mathematical Society 2014

References

[AF] Anderson, F.W. and Fuller, K. R., Rings and categories of modules. Second ed., Graduate Texts in Mathematics, 13, Springer-Verlag, New York, 1992.Google Scholar
[Ar] Artin, E., Galois theory. Dover, New York, 1998.Google Scholar
[ARS] Auslander, M., Reiten, I., and Smalø, S. O., Representation theory of Artin algebras. Cambridge Studies in Advanced Mathematics, 36, Cambridge University Press, Cambridge, 1995.Google Scholar
[BM] Becker, M. F. and MacLane, S., The minimum number of generators for inseparable algebraic extensions.Bull. Amer. Math. Soc. 46 (1940) 182–186. http://dx.doi.org/10.1090/S0002-9904-1940-07169-1 Google Scholar
[B] Bourbaki, N., Algebra II, Springer-Verlag, Berlin, 1990.Google Scholar
[BDS] Browkin, J., Diviš, B., and Schinzel, A., Addition of sequences in general fields.Monatsh. Math. 82 (1976), no. 4, 261–268. http://dx.doi.org/10.1007/BF01540597 Google Scholar
[CS1] Cagliero, L. and Szechtman, F., The classification of uniserial sl(2) nV(m)-modules and a new interpretation of the Racah-Wigner 6 j-symbol.J. Algebra 386 (2013) 142–175. http://dx.doi.org/10.1016/j.jalgebra.2013.03.022 Google Scholar
[CS2] Cagliero, L. and Szechtman, F., Classification of linked indecomposable modules of a family of solvable Lie algebras over an arbitrary field of characteristic 0. arxiv:1407.8125Google Scholar
[DF] Dummit, D. S. and Foote, R. M., Abstract algebra. Third ed., JohnWiley & Sons, Hoboken, NJ, 2004.Google Scholar
[DK] Drozd, Y. and Kirichenko, V., Finite-dimensional algebras. Springer-Verlag, Berlin, 1994.Google Scholar
[EG] Eisenbud, D. and Griffith, P., Serial rings.J. Algebra 17 (1971), 389–400. http://dx.doi.org/10.1016/0021-8693(71)90020-2 Google Scholar
[Fa] Facchini, A., Module theory. Endomorphism rings and direct sum decompositions in some classes of modules. Progress in Mathematics, 167, Birkhäuser, Basel, 1998.Google Scholar
[GP] Gelfand, I. M. and Ponomarev, V. A., Remarks on the classification of a pair of commuting linear transformations in a finite dimensional vector space.Functional Anal. Appl. 3 (1969), 325–326. http://dx.doi.org/10.1007/BF01674013 Google Scholar
[GS] Guerszenzvaig, N. and Szechtman, F., Generalized Artin-Schreier polynomials. arxiv:1306.3967Google Scholar
[I] Isaacs, I. M., Degrees of sums in a separable field extension.Proc. Amer. Math. Soc. 25 (1970), 638–641. http://dx.doi.org/10.1090/S0002-9939-1970-0258803-3 Google Scholar
[K] Kaplansky, I., Fields and rings. The University of Chicago Press, Chicago, IL, 1969.Google Scholar
[M] McCarthy, P. J., Algebraic extensions of fields. Dover, New York, 1991.Google Scholar
[Ma] Makedonskyi, I., On wild Lie algebras. arxiv:1202.1401v2Google Scholar
[N1] Nagell, T., Bemerkungen über zusammengesetzte Zahlkörper, Avh. Norske Vid. Akad. Oslo (1937), 1–26.Google Scholar
[N2] Nagell, T., Bestimmung des Grades gewisser relativalgebraischer Zahlen.Monatsh. Math. Phys. 48 (1939) 61–74.Google Scholar
[Na] Nakayama, T., On Frobeniusean algebras. II. Ann. of Math. 42 (1941), 1–21. http://dx.doi.org/10.2307/1968984 Google Scholar
[P] Petrenko, B. V., On the sum of two primitive elements of maximal subfields of a finite field.Finite Fields Appl. 9 (2003), no. 1, 102–116. http://dx.doi.org/10.1016/S1071-5797(02)00014-X Google Scholar
[Pu] Puninski, G., Serial rings. Kluwer Academic Publishers, Dordrecht, 2001.Google Scholar
[R] Robinson, D. J. S., A course in the theory of groups. Graduate Texts in Mathematics, 80, Springer-Verlag, New York, 1996.Google Scholar
[SL] Shores, T. andLewis, W., Serial modules and endomorphism rings.Duke Math. J. 41 (1974), 889–909. http://dx.doi.org/10.1215/S0012-7094-74-04188-X Google Scholar
[Sr] Srinivasan, B., On the indecomposable representations of a certain class of groups.Proc. London Math. Soc. 10 (1960), 497–513. http://dx.doi.org/10.1112/plms/s3-10.1.497 Google Scholar
[St] Steinitz, E., Algebraische Theorie der Körper.J. Reine Angew. Math. 137 (1910), 167–309.Google Scholar
[T] Teichmüller, O., p-Algebren.Deutsche Mathematik, 1 (1936), 362–388.Google Scholar
[W] Weintraub, S. H., Observations on primitive, normal, and subnormal elements of field extensions.Monatsh. Math. 162 (2011) no. 2, 239–244. http://dx.doi.org/10.1007/s00605-009-0147-6 Google Scholar