Let 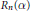${{R}_{n}}\left( \alpha \right)$ be the $n!\,\times \,n!$ matrix whose matrix elements 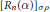${{\left[ {{R}_{n}}\left( \alpha \right) \right]}_{\sigma p}}$ , with $\sigma$ and $p$ in the symmetric group 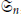${{G}_{n}}$ , are ${{\alpha }^{\ell \left( \sigma {{p}^{-1}} \right)}}$ with 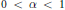$0\,<\,\alpha \,<\,1$, where $\ell \left( \text{ }\!\!\pi\!\!\text{ } \right)$ denotes the number of cycles in 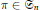$\text{ }\pi \text{ }\in {{G}_{n}}.$ We give the spectrum of ${{R}_{n}}$ and show that the ratio of the largest eigenvalue ${{\text{ }\!\!\lambda\!\!\text{ }}_{0}}$ to the second largest one (in absolute value) increases as a positive power of $n$ as 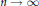$n\,\to \,\infty$.