Analogues of van der Waerden’s theorem on arithmetic progressions are considered where the family of all arithmetic progressions, $\text{AP}$, is replaced by some subfamily of $\text{AP}$. Specifically, we want to know for which sets 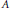$A$, of positive integers, the following statement holds: for all positive integers $r$ and $k$, there exists a positive integer 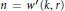$n={w}'\text{(}k,r)$ such that for every $r$-coloring of 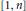$[1,\,n]$ there exists a monochromatic $k$-term arithmetic progression whose common difference belongs to 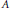$A$. We will call any subset of the positive integers that has the above property large. A set having this property for a specific fixed $r$ will be called $r$-large. We give some necessary conditions for a set to be large, including the fact that every large set must contain an infinite number of multiples of each positive integer. Also, no large set 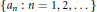$\{{{a}_{n}}\,:\,n\,=\,1,\,2,\ldots \}$ can have $\underset{n\to \infty }{\mathop{\lim \,\inf }}\,\,\frac{{{a}_{n+1}}}{{{a}_{n}}}\,>\,1$. Sufficient conditions for a set to be large are also given. We show that any set containing $n$-cubes for arbitrarily large $n$, is a large set. Results involving the connection between the notions of “large” and “2-large” are given. Several open questions and a conjecture are presented.