Hostname: page-component-cd9895bd7-jkksz Total loading time: 0 Render date: 2024-12-23T01:24:52.651Z Has data issue: false hasContentIssue false
  • English
  • Français

On the Chow Groups of Supersingular Varieties

Published online by Cambridge University Press:  20 November 2018

Najmuddin Fakhruddin
Najmuddin Fakhruddin*
Affiliation:
School of Mathematics, Tata Institute of Fundamental Research, Homi Bhabha Road, Mumbai 400005, India, e-mail: [email protected]
Rights & Permissions [Opens in a new window]

Abstract

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

We compute the rational Chow groups of supersingular abelian varieties and some other related varieties, such as supersingular Fermat varieties and supersingular 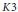$K3$ surfaces. These computations are concordant with the conjectural relationship, for a smooth projective variety, between the structure of Chow groups and the coniveau filtration on the cohomology.

Keywords

14C2514K99
Type
Research Article
Information
Canadian Mathematical Bulletin , Volume 45 , Issue 2 , 01 June 2002 , pp. 204 - 212
Copyright
Copyright © Canadian Mathematical Society 2002

References

[1] Artin, M., Supersingular K3 surfaces. Ann. Sci. École Norm. Sup. 7 (1974), 7–1974.Google Scholar
[2] Beauville, A., Sur l’anneau de Chow d’une variété abélienne. Math. Ann. 273 (1986), 273–1986.Google Scholar
[3] Bloch, S., Torsion algebraic cycles and a theorem of Roitman. Compositio Math. 39 (1979), 39–1979.Google Scholar
[4] Bloch, S., Kas, A. and Lieberman, D., Zero cycles on surfaces with pg = 0. Compositio Math. 33 (1976), 33–1976.Google Scholar
[5] Bloch, S. and Srinivas, V., Remarks on correspondences and algebraic cycles. Amer. J. Math. 105 (1983), 105–1983.Google Scholar
[6] Fulton, W. and Harris, J., Representation Theory. Springer-Verlag, 1991.Google Scholar
[7] Gillet, H., Riemann-Roch theorems for higher algebraic K-theory. Adv. Math. 40 (1981), 40–1981.Google Scholar
[8] Jannsen, U., Motivic sheaves and filtrations on Chow groups. Proc. Symp. Pure Math. 55, Part 1, Amer. Math. Soc, 1994, 245–302.Google Scholar
[9] Manin, Y., Correspondences, motifs and monoidal transformations. Mat. Sb. 6 (1968), 6–1968.Google Scholar
[10] Maruyama, N. and Suwa, N., Remarques sur un article de Bloch et Srinivas sur les cycles algébriques. J. Fac. Sci. Univ. Tokyo 35 (1988), 35–1988.Google Scholar
[11] Murre, J., Applications of algebraic K-theory to the theory of algebraic cycles. Algebraic geometry (Sitges 1983), Springer Lecture Notes in Math. 1124 (1985), 1124–1985.Google Scholar
[12] Murre, J., On a conjectural filtration on the Chow groups of an algebraic variety. Indag. Math. 4 (1993), 4–1993.Google Scholar
[13] Ogus, A., A crystalline Torelli theorem for supersingular K3 surfaces. In: Arithmetic and Geometry, Vol. 2, Progr. Math. 36, Birkhauser, 1983, 361–394.Google Scholar
[14] Oort, F., Subvarieties of moduli spaces. Invent. Math. 24 (1974), 24–1974.Google Scholar
[15] Rudakov, A. N. and Shafarevich, I. R., Surfaces of type K3 over fields of finite characteristic. In: Current problems in mathematics, Vol. 18, Akad. Nauk SSSR, 1981, 115–207.Google Scholar
[16] Saito, H., Abelian varieties attached to cycles of intermediate dimension. NagoyaMath. J. 75 (1979), 75–1979.Google Scholar
[17] Shioda, T. and Katsura, T., On Fermat varieties. Tohoku Math. J. 31 (1979), 31–1979.Google Scholar
[18] Soulé, C., Groupes de Chow et K-théorie de variétés sur un corps fini. Math. Ann. 268 (1984), 268–1984.Google Scholar
[19] Suwa, N., Sur l’image de l’application d’Abel-Jacobi de Bloch. Bull. Soc. Math. France 116 (1988), 116–1988.Google Scholar