Published online by Cambridge University Press: 20 November 2018
The Bezdek–Pach conjecture asserts that the maximum number of pairwise touching positive homothetic copies of a convex body in 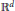 ${{\mathbb{R}}^{d}}$ is
${{\mathbb{R}}^{d}}$ is 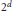 ${{2}^{d}}$. Naszódi proved that the quantity in question is not larger than
${{2}^{d}}$. Naszódi proved that the quantity in question is not larger than 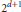 ${{2}^{d+1}}$. We present an improvement to this result by proving the upper bound
${{2}^{d+1}}$. We present an improvement to this result by proving the upper bound 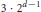 $3\,\cdot \,{{2}^{d-1}}$ for centrally symmetric bodies. Bezdek and Brass introduced the one-sided Hadwiger number of a convex body. We extend this definition, prove an upper bound on the resulting quantity, and show a connection with the problem of touching homothetic bodies.
$3\,\cdot \,{{2}^{d-1}}$ for centrally symmetric bodies. Bezdek and Brass introduced the one-sided Hadwiger number of a convex body. We extend this definition, prove an upper bound on the resulting quantity, and show a connection with the problem of touching homothetic bodies.