Hostname: page-component-cd9895bd7-p9bg8 Total loading time: 0 Render date: 2024-12-25T13:44:18.168Z Has data issue: false hasContentIssue false
  • English
  • Français

On Quasisimilarity for Subnormal Operators, II

Published online by Cambridge University Press:  20 November 2018

John B. Conway
John B. Conway*
Affiliation:
Indiana University, Bloomington Indiana47405
Rights & Permissions [Opens in a new window]

Abstract

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

Let S be a subnormal operator and let 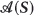be the weak-star closed algebra generated by S and 1. An example of an irreducible cyclic subnormal operator S is found such that there is a T in 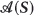with S and T quasisimilar but not unitarily equivalent. However, if S is the unilateral shift, T ∈ 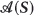and S and T are quasisimilar, then S ≅ T.

Keywords

47B2047B35subnormal operatorToeplitz operatorquasisimilarity
Type
Research Article
Information
Canadian Mathematical Bulletin , Volume 25 , Issue 1 , 01 March 1982 , pp. 37 - 40
Copyright
Copyright © Canadian Mathematical Society 1982

References

1. Clary, W. S., Equality of spectra of quasisimilar hyponormal operators, Proc. Amer. Math. Soc, 53 (1975), 88-90.Google Scholar
2. Clary, W. S., Quasisimilarity and subnormal operators, Ph.D. thesis, University of Michigan, 1973.Google Scholar
3. Conway, J. B., On quasisimilarity for subnormal operators, Illinois, J. Math., 24 (1980), 689-702.Google Scholar
4. Conway, J. B. and Olin, R. F., A functional calculus for subnormal operators, II, Memoirs Amer. Math. Soc, vol. 184, 1977.Google Scholar
5. Halmos, P. R., A Hilbert space problem book, D. Van Nostrand Co., Princeton, 1967.Google Scholar
6. Hastings, W. W., Subnormal operators quasisimilar to an isometry, Trans. Amer. Math. Soc, 256 (1979), 145-161.Google Scholar
7. Hoffman, K., Banach spaces of analytic functions, Prentice-Hall, Englewood Cliffs, N.J. (1962).Google Scholar
8. Wogen, W. R., On some operators with cyclic vectors, Indiana Univ. Math. J., 27 (1978), 163-171.Google Scholar