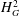 $H_{G}^{2}$
$H_{G}^{2}$Published online by Cambridge University Press: 06 December 2019
We give a characterization of all Parseval wavelet frames arising from a given frame multiresolution analysis. As a consequence, we obtain a description of all Parseval wavelet frames associated with a frame multiresolution analysis. These results are based on a version of Oblique Extension Principle with the assumption that the origin is a point of approximate continuity of the Fourier transform of the involved refinable functions. Our results are written for reducing subspaces.