Hostname: page-component-669899f699-8p65j Total loading time: 0 Render date: 2025-04-24T12:20:49.866Z Has data issue: false hasContentIssue false
  • English
  • Français

On Mutually m-permutable Products of Smooth Groups

Published online by Cambridge University Press:  20 November 2018

A. M. Elkholy  and
M. H. Abd El-Latif
A. M. Elkholy
Affiliation:
Beni Suef University, Faculty of Science, Mathematics Department, Beni-Suef 62511, Egypt e-mail: [email protected]
M. H. Abd El-Latif
Affiliation:
Beni Suef University, Faculty of Science, Mathematics Department, Beni-Suef 62511, Egypt e-mail: [email protected]
Rights & Permissions [Opens in a new window]

Abstract

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

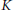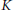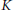Let $G$ be a finite group and $H$, $K$ two subgroups of $G$. A group $G$ is said to be a mutually $m$-permutable product of $H$ and $K$ if $G\,=\,HK$ and every maximal subgroup of $H$ permutes with $K$ and every maximal subgroup of 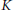$K$ permutes with $H$. In this paper, we investigate the structure of a finite group that is a mutually $m$-permutable product of two subgroups under the assumption that its maximal subgroups are totally smooth.

Keywords

20D1020D2020E1520F16permutable subgroupsm-permutablesmooth groupssubgroup lattices
Type
Research Article
Information
Canadian Mathematical Bulletin , Volume 57 , Issue 2 , 14 June 2014 , pp. 277 - 282
Copyright
Copyright © Canadian Mathematical Society 2014

References

[1] Asaad, M., A condition for the supersolvability of finite groups. Comm. Algebra 38 (2010, no. 10, 3616–3620.http://dx.doi.org/10.1080/00927870903200927 CrossRefGoogle Scholar
[2] Ballester-Bolinches, A., Cossey, J., and Pedraza-Aguilera, M. C., On the products of finite supersolvable groups. Comm. Algebra 29 (2001 , no. 7, 3145–3152. http://dx.doi.org/10.1081/AGB-5013 CrossRefGoogle Scholar
[3] Elkholy, A. M., On totally smooth groups. Int. J. Algebra 1 (2007 , no. 1–4, 63–70.CrossRefGoogle Scholar
[4] Ore, O., Contributions to the theory of groups of finite order. Duke Math. J. 5 (1939 , 431–460.http://dx.doi.org/10.1215/S0012-7094-39-00537-5 CrossRefGoogle Scholar
[5] Schmidt, R., Smooth groups. Geom. Dedicata 84 (2001 , no. 1–3. 183–206.http://dx.doi.org/10.1023/A:1010333719254 CrossRefGoogle Scholar
[6] Schmidt, R., Subgroup lattices of groups. Walter de Gruyter, Berlin, 1994.Google Scholar