No CrossRef data available.
Published online by Cambridge University Press: 20 November 2018
It is known that the Toeplitz algebra associated with any flow which is both minimal and uniquely ergodic always has a trivial 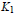 ${{K}_{1}}$-group. We show in this note that if the unique ergodicity is dropped, then such
${{K}_{1}}$-group. We show in this note that if the unique ergodicity is dropped, then such 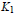 ${{K}_{1}}$-group can be non-trivial. Therefore, in the general setting of minimal flows, even the
${{K}_{1}}$-group can be non-trivial. Therefore, in the general setting of minimal flows, even the 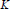 $K$-theoretical index is not sufficient for the classification of Toeplitz operators which are invertible modulo the commutator ideal.
$K$-theoretical index is not sufficient for the classification of Toeplitz operators which are invertible modulo the commutator ideal.