Hostname: page-component-cd9895bd7-gxg78 Total loading time: 0 Render date: 2024-12-29T02:41:29.756Z Has data issue: false hasContentIssue false
  • English
  • Français

On Carleman Integral Operators

Published online by Cambridge University Press:  20 November 2018

Charles G. Costley
Charles G. Costley*
Affiliation:
McGill University, Montreal, Quebec
Rights & Permissions [Opens in a new window]

Extract

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

L2(a, b)

1

with the property

2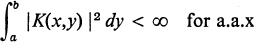

were originally defined by T. Carleman [4]. Here he imposed on the kernel the conditions of measurability and hermiticity,

3

for all x with the exception of a countable set with a finite number of limit points and

4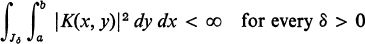

where Jδ denotes the interval [a, b] with the exception of subintervals |x - ξv| < δ; here ξv represents a finite set of points for which (3) fails to hold.

Type
Research Article
Information
Canadian Mathematical Bulletin , Volume 13 , Issue 3 , September 1970 , pp. 351 - 357
Copyright
Copyright © Canadian Mathematical Society 1970

References

1. Achiezer, N. I., Upschi Math. Nauk. 5 (1947), (21) (93).Google Scholar
2. Stone, M. H., Linear transformations in Hilbert space and their applications to analysis, American Math. Soc. Colloq. Publ., Vol. XV, New York, 1932.Google Scholar
3. Korotkov, V. B., Integral operators with Carleman kernels, Differencial'nye Uravnenija 2, (1965), 252-265. (Math. Reviews Vol. 32 (1966), Part 2).Google Scholar
4. Carleman, T., Sur les équations intégrales singulières à noyau reél et symétrique, Uppsala 1923.Google Scholar
5. Carleman, T., La théorie des équations intégrales singulières et ses applications, Ann. Inst. H. Poincaré, 1931.Google Scholar
6. Riesz, M. F., Uber système integrierbarer Funktionen, Math. Ann., 1910.Google Scholar
7. Targonski, G. I., Seminar on functional operators and equations. Lecture Notes in Mathematics No. 33, Springer-Verlag, Berlin, 1962.Google Scholar
8. Misra, B., Speiser, D., and Targonski, Gy., Helv, Phys. Acta 36 (1963), 963-980.Google Scholar