Published online by Cambridge University Press: 20 November 2018
In an attempt to resolve a folklore conjecture of Erdös regarding the non-vanishing at  $s\,=\,1$ of the
$s\,=\,1$ of the  $L$-series attached to a periodic arithmetical function with period
$L$-series attached to a periodic arithmetical function with period  $q$ and values in
$q$ and values in 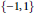 $\left\{ -1,\,1 \right\}$, Livingston conjectured the
$\left\{ -1,\,1 \right\}$, Livingston conjectured the 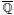 $\overline{\mathbb{Q}}$-linear independence of logarithms of certain algebraic numbers. In this paper, we disprove Livingston’s conjecture for composite
$\overline{\mathbb{Q}}$-linear independence of logarithms of certain algebraic numbers. In this paper, we disprove Livingston’s conjecture for composite 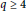 $q\,\ge \,4$, highlighting that a new approach is required to settle Erdös conjecture. We also prove that the conjecture is true for prime
$q\,\ge \,4$, highlighting that a new approach is required to settle Erdös conjecture. We also prove that the conjecture is true for prime 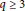 $q\,\ge \,3$, and indicate that more ingredients will be needed to settle Erdös conjecture for prime
$q\,\ge \,3$, and indicate that more ingredients will be needed to settle Erdös conjecture for prime  $q$.
$q$.