Let GF(q) denote the finite field with q = pn elements and let

(1)

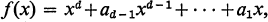
where each ai ∊ GF(q) and 1 < d <p. For r=2, 3, …, d we let nr denote the number of solutions (x1, …, xr) over GF(q) of

(2)

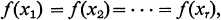
for which x1, …, xr are all different. Birch and Swinnerton-Dyer [1] have shown that

(3)

where each vr is a nonnegative integer depending on f and q and the constant implied by the O-symbol depends here, and throughout the paper, only on d.