No CrossRef data available.
Article contents
A Note on Detecting Algebraic Cycles
Published online by Cambridge University Press: 20 November 2018
Abstract
The purpose of this note is to show that the homologically trivial cycles contructed by Clemens and their generalisations due to Paranjape can be detected by the technique of spreading out. More precisely, we associate to these cycles (and the ambient varieties in which they live) certain families which arise naturally and which are defined over 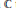 $\mathbb{C}$ and show that these cycles, along with their relations, can be detected in the singular cohomology of the total space of these families.
$\mathbb{C}$ and show that these cycles, along with their relations, can be detected in the singular cohomology of the total space of these families.
Keywords
- Type
- Research Article
- Information
- Copyright
- Copyright © Canadian Mathematical Society 2006


