Published online by Cambridge University Press: 20 November 2018
A ring R (associative ring) is said to be fully ordered provided that R is a linearly ordered set under a relation 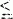 such that for any a, b and c in R,
such that for any a, b and c in R, 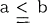 implies that
implies that 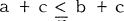 and if c ε 0 then
and if c ε 0 then 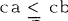 and
and 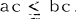 . We say a subset K of R is convex provided that if a, b ε K such that
. We say a subset K of R is convex provided that if a, b ε K such that  then the interval [a, b] is a subset of K. Obviously an additive subgroup K of R is convex if and only if b ε K and b > 0 implies [a, b] ⊆ K.
then the interval [a, b] is a subset of K. Obviously an additive subgroup K of R is convex if and only if b ε K and b > 0 implies [a, b] ⊆ K.