Hostname: page-component-7479d7b7d-t6hkb Total loading time: 0 Render date: 2024-07-08T16:54:54.333Z Has data issue: false hasContentIssue false
  • English
  • Français

Non-Isomorphic Maximal Orders with Isomorphic Matrix Rings

Published online by Cambridge University Press:  20 November 2018

A. W. Chatters
A. W. Chatters*
Affiliation:
School of Mathematics, University of Bristol, University Walk, Bristol BS8 1TW, UK, email: [email protected]
Rights & Permissions [Opens in a new window]

Abstract

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

We construct a countably infinite family of pairwise non-isomorphic maximal 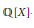$\mathbb{Q}\left[ X \right]$-orders such that the full 2 by 2 matrix rings over these orders are all isomorphic.

Keywords

16S5016H0516N60
Type
Research Article
Information
Canadian Mathematical Bulletin , Volume 43 , Issue 4 , 01 December 2000 , pp. 413 - 417
Copyright
Copyright © Canadian Mathematical Society 2000

References

[1] Chatters, A. W., Non-isomorphic rings with isomorphic matrix rings. Proc. Edinburgh Math. Soc. 36 (1993), 339–348.Google Scholar
[2] Chatters, A. W., Matrix-isomorphic maximal ℤ-orders. J. Algebra 181 (1996), 593–600.Google Scholar
[3] Swan, R. G., Projective modules over group rings and maximal orders. Ann. of Math. 76 (1962), 55–61.Google Scholar
[4] Webber, D. B., Ideals and modules of simple Noetherian hereditary rings. J. Algebra 16 (1970), 239–242.Google Scholar