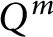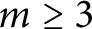Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by Crossref.
Li, Dehe
and
Zhai, Shujie
2021.
Real Hypersurfaces in Complex Grassmannians of Rank Two.
Mathematics,
Vol. 9,
Issue. 24,
p.
3238.
Hu, Zejun
Yao, Zeke
and
Zhang, Xi
2021.
On Hopf hypersurfaces of the complex quadric with recurrent Ricci tensor.
Journal of Geometry,
Vol. 112,
Issue. 3,
Zhang, Xi
Gao, Dong
Hu, Zejun
and
Yao, Zeke
2023.
On Hopf hypersurfaces of S2×S2 and H2×H2.
Journal of Geometry and Physics,
Vol. 194,
Issue. ,
p.
104996.
Hu, Zejun
and
Zhang, Xi
2024.
On some hypersurfaces of $${\mathbb {S}}^2\times {\mathbb {S}}^2$$ and $${\mathbb {H}}^2\times {\mathbb {H}}^2$$.
Revista de la Real Academia de Ciencias Exactas, Físicas y Naturales. Serie A. Matemáticas,
Vol. 118,
Issue. 3,