The study carried out in this paper about some new examples of Banach spaces, consisting of certain valued fields extensions, is a typical non-archimedean feature. We determine whether these extensions are of countable type, have $t$-orthogonal bases, or are reflexive. As an application we construct, for a class of base fields, a norm 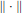$\left\| \,.\, \right\|$ on ${{c}_{0}}$, equivalent to the canonical supremum norm, without non-zero vectors that are 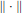$\left\| \,.\, \right\|$-orthogonal and such that there is a multiplication on ${{c}_{0}}$ making 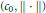$\left( {{c}_{0}},\,\left\| \,.\, \right\| \right)$ into a valued field.