Hostname: page-component-586b7cd67f-2brh9 Total loading time: 0 Render date: 2024-11-25T05:06:16.156Z Has data issue: false hasContentIssue false
  • English
  • Français

A Method of Solving a Class of CIV Boundary Value Problems

Published online by Cambridge University Press:  20 November 2018

Nezam Iraniparast
Nezam Iraniparast*
Affiliation:
Western Kentucky University Bowling Green, Kentucky U.S.A. 42101
Rights & Permissions [Opens in a new window]

Abstract

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

A method will be introduced to solve problems utt — uss = h(s, t), u(t,t) - u(1+t,1 - t), u(s,0) = g(s), u(1,1) = 0 and 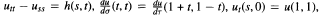for (s, t) in the characteristic triangle R = {(s,t) : t ≤ s ≤ 2 — t, 0 ≤ t ≤ 1}. Here 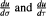represent the directional derivatives of u in the characteristic directions e1 = (— 1, — 1) and e2 = (1, — 1), respectively. The method produces the symmetric Green's function of Kreith [1] in both cases.

Keywords

35L20(35P05)
Type
Research Article
Information
Canadian Mathematical Bulletin , Volume 35 , Issue 3 , 01 September 1992 , pp. 371 - 375
Copyright
Copyright © Canadian Mathematical Society 1992

References

1. Kreith, K., Symmetric Green's Functions for a Class of CTV Boundary Value Problems, Canad. Math. Bull. (3) 31(1988), 272–279.Google Scholar
2. Riesz, F. and Nagy, B. Sz., Functional Analysis , Ungar, New York, 1955.Google Scholar