No CrossRef data available.
Article contents
Linear Relations Among the Values of Canonical Heights from the Existence of Non-Trivial Endomorphisms
Published online by Cambridge University Press: 20 November 2018
Abstract
Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.
We study the interplay between canonical heights and endomorphisms of an abelian variety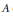 
$A$ over a number field 
$k$. In particular we show that whenever the ring of endomorphisms defined over $k$ is strictly larger than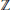 
$\mathbb{Z}$ there will be 
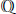$\mathbb{Q}$-linear relations among the values of a canonical height pairing evaluated at a basis modulo torsion of 
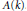$A(k)$.
- Type
- Research Article
- Information
- Copyright
- Copyright © Canadian Mathematical Society 2004
References
[FLSSSW]
Flynn, E., Leprévost, F., Schaefer, E., Stein, W., Stoll, M. and Wetherell, J., Empirical evidence for the Birch and Swinnerton-Dyer conjectures for modular jacobians of genus 2 curves. Math. Comp. (236) 70 (2001), 1675–1697.Google Scholar
[Mi2]
Milne, J. S., On the Arithmetic of Abelian Varieties. Invent.Math. 17 (1972), 177–190.Google Scholar
[Mu]
Mumford, D., Abelian Varieties. Tata Inst. Fund. Res. Stud. Math. 5, Oxford University Press, 1974.Google Scholar
[Ne]
Néron, A., Quasi-fonctions et Hauteurs sur les variétés abéliennes. Ann. of Math. 82 (1965), 249–331.Google Scholar
[Se]
Serre, J.-P., Lectures on the Mordell-Weil Theorem. Aspects Math. E15, Vieweg, Braunschweig, 1989.Google Scholar
[Ta]
Talamanca, V., A note on height pairings on polarized abelian varieties. Atti Accad. Naz. Lincei Rend. Cl. Sci. Fis. Mat. Natur. 10 (1999), 57–60.Google Scholar
You have
Access