Article contents
Higher Moments of Fourier Coefficients of Cusp Forms
Published online by Cambridge University Press: 20 November 2018
Abstract
Let 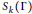 ${{S}_{k}}\left( \Gamma \right)$ be the space of holomorphic cusp forms of even integral weight
${{S}_{k}}\left( \Gamma \right)$ be the space of holomorphic cusp forms of even integral weight 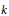 $k$ for the full modular group
$k$ for the full modular group 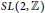 $SL\left( 2,\mathbb{Z} \right)$. Let
$SL\left( 2,\mathbb{Z} \right)$. Let 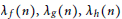 ${{\text{ }\!\!\lambda\!\!\text{ }}_{f}}\left( n \right),{{\text{ }\!\!\lambda\!\!\text{ }}_{g}}\left( n \right),{{\text{ }\!\!\lambda\!\!\text{ }}_{h}}\left( n \right)$ be the
${{\text{ }\!\!\lambda\!\!\text{ }}_{f}}\left( n \right),{{\text{ }\!\!\lambda\!\!\text{ }}_{g}}\left( n \right),{{\text{ }\!\!\lambda\!\!\text{ }}_{h}}\left( n \right)$ be the  $n$-th normalized Fourier coefficients of three distinct holomorphic primitive cusp forms
$n$-th normalized Fourier coefficients of three distinct holomorphic primitive cusp forms 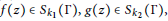 $f\left( z \right)\in {{S}_{{{k}_{1}}}}\left( \Gamma \right),g\left( z \right)\in {{S}_{{{k}_{2}}}}\left( \Gamma \right)$, and
$f\left( z \right)\in {{S}_{{{k}_{1}}}}\left( \Gamma \right),g\left( z \right)\in {{S}_{{{k}_{2}}}}\left( \Gamma \right)$, and  $h\left( z \right)\in {{S}_{{{k}_{3}}}}\left( \Gamma \right)$, respectively. In this paper we study the cancellations of sums related to arithmetic functions, such as
$h\left( z \right)\in {{S}_{{{k}_{3}}}}\left( \Gamma \right)$, respectively. In this paper we study the cancellations of sums related to arithmetic functions, such as 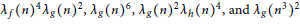 $\text{ }{{\lambda }_{f}}{{\left( n \right)}^{4}}{{\lambda }_{g}}{{\left( n \right)}^{2}},\text{ }{{\lambda }_{g}}{{\left( n \right)}^{6}},\text{ }{{\lambda }_{g}}{{\left( n \right)}^{2}}{{\lambda }_{h}}{{\left( n \right)}^{4}}$, and
$\text{ }{{\lambda }_{f}}{{\left( n \right)}^{4}}{{\lambda }_{g}}{{\left( n \right)}^{2}},\text{ }{{\lambda }_{g}}{{\left( n \right)}^{6}},\text{ }{{\lambda }_{g}}{{\left( n \right)}^{2}}{{\lambda }_{h}}{{\left( n \right)}^{4}}$, and 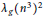 ${{\text{ }\!\!\lambda\!\!\text{ }}_{g}}{{\left( {{n}^{3}} \right)}^{2}}$ twisted by the arithmetic function
${{\text{ }\!\!\lambda\!\!\text{ }}_{g}}{{\left( {{n}^{3}} \right)}^{2}}$ twisted by the arithmetic function 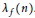 ${{\text{ }\!\!\lambda\!\!\text{ }}_{f}}\left( n \right)$.
${{\text{ }\!\!\lambda\!\!\text{ }}_{f}}\left( n \right)$.
Keywords
- Type
- Research Article
- Information
- Copyright
- Copyright © Canadian Mathematical Society 2015
References
- 3
- Cited by


