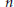Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by Crossref.
Di Natale, Carmelo
and
Holstein, Julian V.S.
2019.
The global derived period map.
Advances in Mathematics,
Vol. 353,
Issue. ,
p.
224.
Levine, Marc
Pepin Lehalleur, Simon
and
Srinivas, Vasudevan
2024.
Euler characteristics of homogeneous and weighted-homogeneous hypersurfaces.
Advances in Mathematics,
Vol. 441,
Issue. ,
p.
109556.