Hostname: page-component-cd9895bd7-jn8rn Total loading time: 0 Render date: 2024-12-23T01:41:38.386Z Has data issue: false hasContentIssue false
  • English
  • Français

Generalized Solution of the Photon Transport Problem

Published online by Cambridge University Press:  20 November 2018

Yu-Hsien Chang  and
Cheng-Hong Hong
Yu-Hsien Chang
Affiliation:
Department of Mathematics, National Taiwan Normal University, Taipei, Taiwan, R. O. C.e-mail: [email protected]@yahoo.com.tw
Cheng-Hong Hong
Affiliation:
Department of Mathematics, National Taiwan Normal University, Taipei, Taiwan, R. O. C.e-mail: [email protected]@yahoo.com.tw
Rights & Permissions [Opens in a new window]

Abstract

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

The purpose of this paper is to show the existence of a generalized solution of the photon transport problem. By means of the theory of equicontinuous 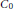${{C}_{0}}$-semigroup on a sequentially complete locally convex topological vector space we show that the perturbed abstract Cauchy problem has a unique solution when the perturbation operator and the forcing term function satisfy certain conditions. A consequence of the abstract result is that it can be directly applied to obtain a generalized solution of the photon transport problem.

Keywords

35K3047D03photon transportC0-semigroup
Type
Research Article
Information
Canadian Mathematical Bulletin , Volume 54 , Issue 1 , 01 March 2011 , pp. 28 - 38
Copyright
Copyright © Canadian Mathematical Society 2011

References

[1] Choe, Y. H., C 0 -semigroups on a locally convex space. J.Math. Anal. Appl. 106(1985), no. 2, 293–320. doi:10.1016/0022-247X(85)90115-5Google Scholar
[2] deLaubenfels, R., Existence families, functional calculi and evolution equations. Lecture Notes in Mathematics, 1570, Springer-Verlag, Berlin, 1994.Google Scholar
[3] Komura, T., Semigroups of operators in locally convex spaces. J. Functional Analysis 2(1968), 258–296. doi:10.1016/0022-1236(68)90008-6Google Scholar
[4] Köthe, G., Topological vector spaces. II. Grundlehren der Mathematischen Wissenschaften, 237, Springer-Verlag, New York-Berlin, 1979.Google Scholar
[5] Lisi, M. and Totaro, S., Photon transport with a localized source in locally convex spaces. Math Methods Appl. Sci. 29(2006), no. 9, 1019–1033. doi:10.1002/mma.713Google Scholar
[6] Lisi, M. and Totaro, S., Inverse problems related to photon transport in an interstellar cloud. Transport Theory Statist. Phys. 32(2003), no. 3–4, 327–345. doi:10.1081/TT-120024767Google Scholar
[7] Teixeira, E. V., Strong solutions for differential equations in abstract spaces. J. Differential Equations 214(2005), no. 1, 65–91. doi:10.1016/j.jde.2004.11.006Google Scholar
[8] Trèves, F., Topological vector spaces, distributions and kernels. Academic Press, New York-London, 1967.Google Scholar
[9] Yosida, K., “Functional analysis,” Academic Press, New York, 1968 Second Edition.Google Scholar