Hostname: page-component-745bb68f8f-l4dxg Total loading time: 0 Render date: 2025-02-11T06:10:23.410Z Has data issue: false hasContentIssue false
  • English
  • Français

Generalized Casimir operators for loop Lie superalgebras

Part of: Special varieties Lie algebras and Lie superalgebras

Published online by Cambridge University Press:  27 January 2025

Abhishek Das  and
Santosha Pattanayak
Abhishek Das
Affiliation:
Department of Mathematics and Statistics, Indian Institute of Technology Kanpur, Kanpur, India [email protected]
Santosha Pattanayak*
Affiliation:
Department of Mathematics and Statistics, Indian Institute of Technology Kanpur, Kanpur, India [email protected]
*
e-mail: [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

Let $\mathfrak{g}$ be the queer superalgebra 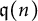$\operatorname {\mathfrak{q}}(n)$ over the field of complex numbers 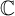$\mathbb C$. For any associative, commutative, and finitely generated 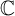$\mathbb C$-algebra A with unity, we consider the loop Lie superalgebra 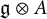$\mathfrak{g} \otimes A$. We define a class of central operators for 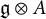$\mathfrak{g} \otimes A$, which generalizes the classical Gelfand invariants. We show that they generate the algebra 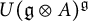$U(\mathfrak{g} \otimes A)^{\mathfrak{g}}$. We also show that there are no non-trivial $\mathfrak{g}$-invariants of $U(\mathfrak{g} \otimes A)$ where 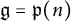$\mathfrak{g}=\mathfrak{p}(n)$, the periplectic Lie superalgebra.

Keywords

Lie superalgebrasCasimir operatorsloop superalgebrasuniversal enveloping algebra

MSC classification

Primary: 17B10: Representations, algebraic theory (weights) 17B35: Universal enveloping (super)algebras 17B65: Infinite-dimensional Lie (super)algebras
Secondary: 14M30: Supervarieties
Type
Article
Information
Canadian Mathematical Bulletin , First View , pp. 1 - 17
Check for updates
Copyright
© The Author(s), 2025. Published by Cambridge University Press on behalf of Canadian Mathematical Society

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

Calixto, L. and Macedo, T., Irreducible modules for equivariant map superalgebras and their extensions . J. Algebra 517(2019), 365–395.Google Scholar
Calixto, L., Moura, A., and Savage, A., Equivariant map queer Lie superalgebras . Can. J. Math. 68(2016), 258–279.Google Scholar
Cheng, S.-J. and Wang, W., Dualities and representations of Lie superalgebras, Graduate Studies in Mathematics, 144, American Mathematical Society, Providence, RI, 2012. MR3012224.Google Scholar
Deligne, P., Lehrer, G. I., and Zhang, R. B., The first fundamental theorem of invariant theory for the orthosymplectic supergroup . Adv. Math. 327(2018), 4–24.Google Scholar
Deligne, P. and Morgan, J. W., Notes on supersymmetry (following Joseph Bernstein) . In: Quantum fields and strings: A course for mathematicians, vol. 1, 2. (Princeton, NJ, 1996/1997), (editors P. Deligne, P. Etingof, D. S. Freed, L. C. Jeffrey, D. Kazhdan, J. W. Morgan, D. R. Morrison, E. Witten), American Mathematical Society, Providence, RI, 1999, pp. 41–97.Google Scholar
Goodman, R. and Wallach, N. R., Symmetry, representations, and invariants, Graduate Texts in Mathematics, 255, Springer, Dordrecht, 2009.Google Scholar
Gorelik, M., The center of a simple $P$ -type Lie superalgebra . J. Algebra 246(2001), 414–428.Google Scholar
Kac, V. G., Lie superalgebras . Adv. Math. 26(1977), 8–96.Google Scholar
Moon, D., Tensor product representations of the Lie superalgebra $\mathfrak{p}(n)$ and their centralizers . Commun. Algebra 31(2003), no. 5, 2095–2140.Google Scholar
Mukherjee, S., Pattanayak, S., and Sharma, S. S., On some central operators for loop Lie superalgebras . J. Pure Appl. Algebra 228(2024), no. 7. https://doi.org/10.1016/j.jpaa.2024.107630.Google Scholar
Musson, I. M., Lie superalgebras and enveloping algebras. Graduate Studies in Mathematics, 131, American Mathematical Society, Providence, RI, 2012.Google Scholar
Rao, S. E., Finite dimensional modules for multiloop superalgebras of types A(m,n) and C(m) . Proc. Am. Math. Soc. 141(2013), no. 10, 3411–3419.Google Scholar
Rao, S. E., Generalized Casimir operators . J. Algebra Appl. 18(2019), no. 11, 1950219.Google Scholar
Rao, S. E., Generalized Casimir operators for Lie superalgebras . J. Math. Phys. 62(2021), 101703.Google Scholar
Rao, S. E. and Zhao, K., On integrable representations for toroidal Lie superalgebras . Contemp. Math. 343(2004), 243–261.Google Scholar
Savage, A., Equivariant map superalgebras . Math. Z. 277(2014), nos. 1–2, 373–399.Google Scholar
Senesi, P., Finite-dimensional representation theory of loop algebras: A survey: Quantum affine algebras, extended affine Lie algebras, and their applications . Contemp. Math. 506(2010), 263–283.Google Scholar
Sergeev, A., The centre of enveloping algebra for Lie superalgebra $Q(n,\mathbb{C})$ . Lett. Math. Phys. 7(1983), no. 3, 177–179.Google Scholar
Sergeev, A., An analog of the classical invariant theory for Lie superalgebras . I. Mich. Math. J. 49(2001), no. 1, 113–146.Google Scholar
Wei, J., Zheng, L., and Shu, B., Centers of universal enveloping algebras of Lie superalgebras in prime characteristic. Preprint, arXiv:1210.8032v4.Google Scholar