Hostname: page-component-cd9895bd7-dk4vv Total loading time: 0 Render date: 2024-12-24T13:48:55.876Z Has data issue: false hasContentIssue false
  • English
  • Français

A Generalization of a Theorem of Boyd and Lawton

Published online by Cambridge University Press:  20 November 2018

Zahraa Issa  and
Matilde Lalín
Zahraa Issa
Affiliation:
Département de mathématiques et de statistique, Université de Montréal, Montreal, QC H3C 3J7 e-mail: [email protected]@dms.umontreal.ca
Matilde Lalín
Affiliation:
Département de mathématiques et de statistique, Université de Montréal, Montreal, QC H3C 3J7 e-mail: [email protected]@dms.umontreal.ca
Rights & Permissions [Opens in a new window]

Abstract.

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

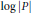The Mahler measure of a nonzero $n$-variable polynomial $P$ is the integral of $\log \,\left| P \right|$ on the unit $n$-torus. A result of Boyd and Lawton says that the Mahler measure of a multivariate polynomial is the limit of Mahler measures of univariate polynomials. We prove the analogous result for different extensions of Mahler measure such as generalized Mahler measure (integrating the maximum of 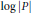$\log \,\left| P \right|$ for possibly different 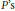${{P}^{'}}\text{s}$), multiple Mahler measure (involving products of 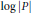$\log \,\left| P \right|$ for possibly different 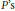${{P}^{'}}\text{s}$), and higher Mahler measure (involving 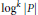${{\log }^{k}}\,\left| P \right|$).

Keywords

11R0611R09Mahler measurepolynomial
Type
Research Article
Information
Canadian Mathematical Bulletin , Volume 56 , Issue 4 , 01 December 2013 , pp. 759 - 768
Copyright
Copyright © Canadian Mathematical Society 2013

References

[BBSW] Borwein, D., Borwein, J., Straub, A., and Wan, J., Log-sine evaluations of Mahler measures. II. Integers, to appear.Google Scholar
[BS] Borwein, J. and Straub, A., Log-sine evaluations of Mahler measures. J. Aust. Math. Soc., to appear.Google Scholar
[Bo81a] Boyd, D.W., Speculations concerning the range of Mahler's measure. Canad. Math. Bull. 24 (1981), no. 4, 453–469. http://dx.doi.org/10.4153/CMB-1981-069-5 http://dx.doi.org/10.4153/CMB-1981-069-5 Google Scholar
[Bo81b] Boyd, D.W., Kronecker's theorem and Lehmer's problem for polynomials in several variables. J. Number Theory 13 (1981), no. 1, 116–121. http://dx.doi.org/10.1016/0022-314X(81)90033-0 http://dx.doi.org/10.1016/0022-314X(81)90033-0 Google Scholar
[EW99] Everest, G. and T.Ward, Heights of polynomials and entropy in algebraic dynamics. Universitext, Springer-Verlag London, Ltd., London, 1999.Google Scholar
[GO04] Gon, Y. and Oyanagi, H., Generalized Mahler measures and multiple sine functions. Internat. J. Math. 15 (2004), no. 5, 425–442. http://dx.doi.org/10.1142/S0129167X04002363 http://dx.doi.org/10.1142/S0129167X04002363 Google Scholar
[KLO08] Kurokawa, N., Lalín, M., and Ochiai, H., Higher Mahler measures and zeta functions. Acta Arith. 135 (2008), no. 3, 269–297. http://dx.doi.org/10.4064/aa135-3-5 http://dx.doi.org/10.4064/aa135-3-5 Google Scholar
[La08] Lalín, M. N., Mahler measures and computations with regulators. J. Number Theory 128 (2008), no. 5, 1231–1271. http://dx.doi.org/10.1016/j.jnt.2007.03.002 http://dx.doi.org/10.1016/j.jnt.2007.03.002 Google Scholar
[La83] Lawton, W. M., A problem of Boyd concerning geometric means of polynomials. J. Number Theory 16 (1983), no. 3, 356–362. http://dx.doi.org/10.1016/0022-314X(83)90063-X http://dx.doi.org/10.1016/0022-314X(83)90063-X Google Scholar
[Le33] Lehmer, D. H., Factorization of certain cyclotomic functions. Ann. of Math. (2) 34 (1933), no. 3, 461–479. http://dx.doi.org/10.2307/1968172 http://dx.doi.org/10.2307/1968172 Google Scholar
[Ma62] Mahler, K., On some inequalities for polynomials in several variables. J. London Math. Soc. 37 (1962), 341–344. http://dx.doi.org/10.1112/jlms/s1-37.1.341 Google Scholar
[Sa10] Sasaki, Y., On multiple higher Mahler measures and multiple L values. Acta Arith. 144 (2010), no. 2, 159–165. http://dx.doi.org/10.4064/aa144-2-5 http://dx.doi.org/10.4064/aa144-2-5 Google Scholar