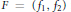Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by Crossref.
Franc, Cameron
and
Mason, Geoffrey
2016.
Hypergeometric series, modular linear differential equations and vector-valued modular forms.
The Ramanujan Journal,
Vol. 41,
Issue. 1-3,
p.
233.
Franc, Cameron
and
Mason, Geoffrey
2016.
Three-dimensional imprimitive representations of the modular group and their associated modular forms.
Journal of Number Theory,
Vol. 160,
Issue. ,
p.
186.
Franc, Cameron
Gannon, Terry
and
Mason, Geoffrey
2018.
On unbounded denominators and hypergeometric series.
Journal of Number Theory,
Vol. 192,
Issue. ,
p.
197.
Candelori, Luca
Hartland, Tucker
Marks, Christopher
and
Yépez, Diego
2018.
Indecomposable vector-valued modular forms and periods of modular curves.
Research in Number Theory,
Vol. 4,
Issue. 2,
Beem, Christopher
and
Rastelli, Leonardo
2018.
Vertex operator algebras, Higgs branches, and modular differential equations.
Journal of High Energy Physics,
Vol. 2018,
Issue. 8,
Bajpai, Jitendra
2019.
Lifting of Modular Forms.
Publications mathématiques de Besançon. Algèbre et théorie des nombres,
p.
5.
Grady, J. Connor
Lam, Ching Hung
Tener, James E.
and
Yamauchi, Hiroshi
2020.
Classification of extremal vertex operator algebras with two simple modules.
Journal of Mathematical Physics,
Vol. 61,
Issue. 5,
Franc, Cameron
and
Mason, Geoffrey
2020.
Classification of some vertex operator algebras of rank 3.
Algebra & Number Theory,
Vol. 14,
Issue. 6,
p.
1613.
Gottesman, Richard
2020.
The arithmetic of vector-valued modular forms on Γ0(2).
International Journal of Number Theory,
Vol. 16,
Issue. 02,
p.
241.
Franc, Cameron
and
Mason, Geoffrey
2020.
Constructions of vector-valued modular forms of rank four and level one.
International Journal of Number Theory,
Vol. 16,
Issue. 05,
p.
1111.
Grabner, Peter J.
2020.
Quasimodular forms as solutions of modular differential equations.
International Journal of Number Theory,
Vol. 16,
Issue. 10,
p.
2233.
Jian, Zhang
and
Fu-Wang, Zhao
2021.
Improved extended Gaussian image and its application on 3D point clouds registration.
Journal of Intelligent & Fuzzy Systems,
p.
1.
Kaidi, Justin
and
Perlmutter, Eric
2021.
Discreteness and integrality in Conformal Field Theory.
Journal of High Energy Physics,
Vol. 2021,
Issue. 2,
Fiori, Andrew
and
Franc, Cameron
2022.
The unbounded denominators conjecture for the noncongruence subgroups of index 7.
Journal of Number Theory,
Vol. 240,
Issue. ,
p.
611.
Calegari, Frank
Dimitrov, Vesselin
and
Tang, Yunqing
2025.
The unbounded denominators conjecture.
Journal of the American Mathematical Society,
Vol. 38,
Issue. 3,
p.
627.