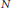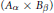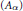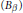Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by Crossref.
Cavaleri, Matteo
2017.
Computability of Følner sets.
International Journal of Algebra and Computation,
Vol. 27,
Issue. 07,
p.
819.
Masquijo, Julio Javier
Allende, Victoria
Porta Alesandria, Julieta
López Villagra, María Belén
and
Paz, Miguel Justo Mariano
2021.
Resultados funcionales del tratamiento de coaliciones calcáneo-escafoideas con resección e interposición de grasa autóloga: Experiencia de dos centros.
Revista de la Asociación Argentina de Ortopedia y Traumatología,
Vol. 86,
Issue. 3,
p.
342.