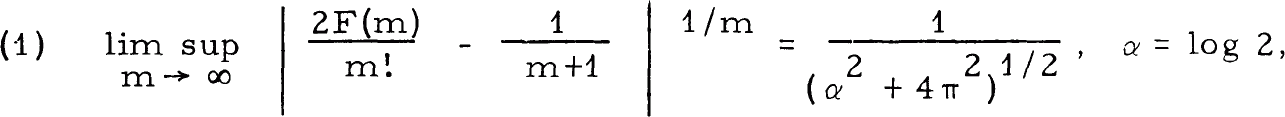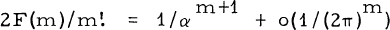Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by Crossref.
Prodinger, Helmut
1983.
Ordered Fibonacci Partitions.
Canadian Mathematical Bulletin,
Vol. 26,
Issue. 3,
p.
312.
Qi, Feng
2019.
Determinantal expressions and recurrence relations for Fubini and Eulerian polynomials.
Journal of Interdisciplinary Mathematics,
Vol. 22,
Issue. 3,
p.
317.
AĞCA, Muhammet
and
ÖZMEN, Nejla
2023.
Miscellaneous Properties of Generalized Fubini Polynomials.
Communications in Advanced Mathematical Sciences,
Vol. 6,
Issue. 1,
p.
19.
Simsek, Yilmaz
and
Kilar, Neslihan
2023.
Exploring Mathematical Analysis, Approximation Theory, and Optimization.
Vol. 207,
Issue. ,
p.
279.