No CrossRef data available.
Published online by Cambridge University Press: 20 November 2018
The purpose of the paper is to extend a tight set function on a lattice 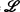 with values in a locally convex space of special type to a measure on the cr-ring generated by
with values in a locally convex space of special type to a measure on the cr-ring generated by 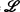 . This result generalizes the extension theorem of Thomas [12, p. 151], which in turn contains the extension theorems of Pauc [9, p. 710], Fox [4, p. 525] and J. J. Uhl, Jr. [14, Corollary 2].
. This result generalizes the extension theorem of Thomas [12, p. 151], which in turn contains the extension theorems of Pauc [9, p. 710], Fox [4, p. 525] and J. J. Uhl, Jr. [14, Corollary 2].